The three-period binomial interest rate tree provided below gives one-period interest rates and prices of zero-coupon bonds. Starting at t = 0, you are provided with the one-period interest rate and prices of zero-coupon bonds with maturities of one period, two periods, three periods, and four periods. At t=1, you are provided with the one-period interest rate and prices of zero-coupon bonds with maturities of one period, two periods, and three periods. At t=2, you are provided with the one-period interest rate and prices of zero-coupon bonds with maturities of one period and two periods. At t=3, you are provided with the one-period interest rate and prices of zero-coupon bonds with maturity of one period. 

Calculate the price of a two-period floor (depicted below by a rectangle) with an exercise rate of 9 percent. The underlying is the one-period rate.

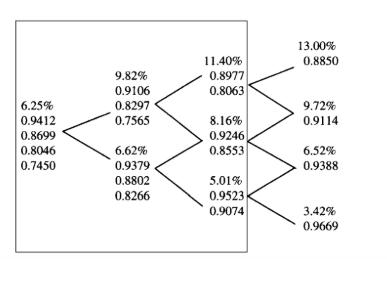
6.25% 0.9412 0.8699 0.8046 0.7450 9.82% 0.9106 0.8297 0.7565 6.62% 0.9379 0.8802 0.8266 11.40% 0.8977 0.8063 8.16% 0.9246 0.8553 5.01% 0.9523 0.9074 13.00% 0.8850 m 9.72% 0.9114 6.52% 0.9388 3.42% 0.9669