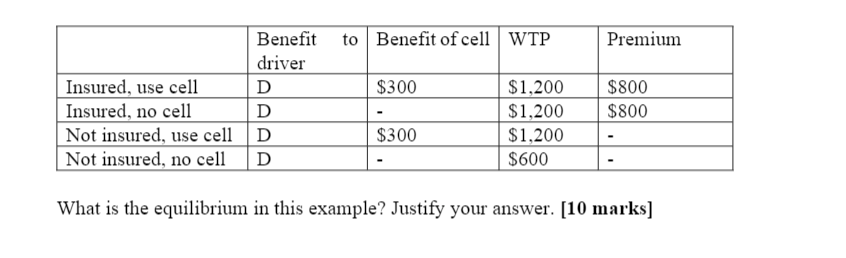
There are 1000 identical people who like to drive while talking on their cell phones. Each driver has a 0.04 probability of one accident each year if she does not use her cell phone and 0.08 probability of one accident each year if she does. Any accident results in $10,000 damages to the driver's car. Assume the insurance market is competitive, companies charge premiums just sufficient to cover expected loses, but the insurance companies cannot tell whether or not the customer uses her cell phone while driving. If nobody uses a cell phone the premium is $400 (0.04*$10,000), if everybody uses their cell phone the premium is $800 (0.08*$10,000); if there is a mix the premium will be within these limits. Drivers like to use their cell phones and derive an additional $300 worth of satisfaction from its convenience. The benefit of driving is an unknown, positive constant, $D. Drivers are risk averse and are WTP $600 per year to insure against a 0.04 probability of a $10,000 accident and $1,200 per year to insure against a 0.08 probability of a $10,000 accident. To summarise:

Benefit to Benefit of cell WTP Premium driver Insured, use cell D $300 $1,200 $800 Insured, no cell D $1,200 $800 Not insured, use cell D $300 $1,200 Not insured, no cell D $600 What is the equilibrium in this example? Justify your answer. [10 marks]