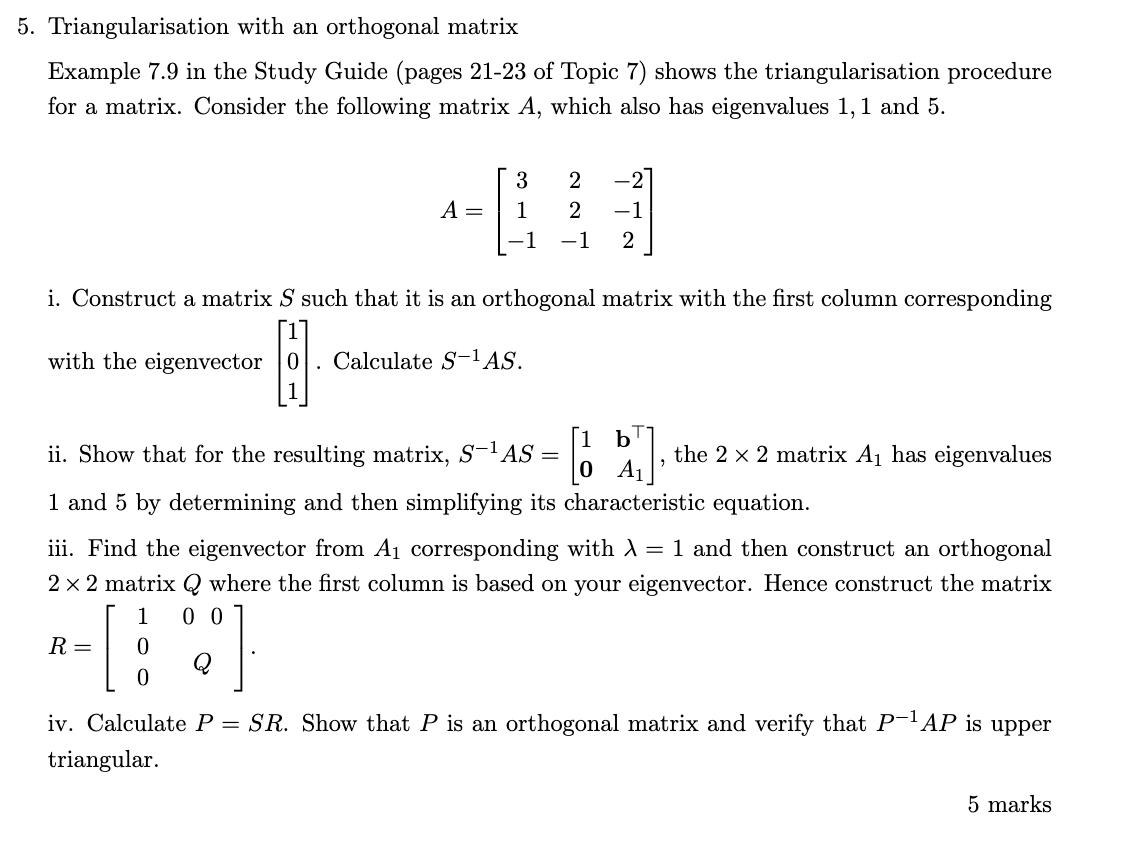
5. Triangularisation with an orthogonal matrix Example 7.9 in the Study Guide (pages 21-23 of Topic 7) shows the triangularisation procedure for a matrix. Consider the following matrix A, which also has eigenvalues 1, 1 and 5. A = . 3 2 1 -1 R = - 2 2 -1 i. Construct a matrix S such that it is an orthogonal matrix with the first column corresponding with the eigenvector 0 A Calculate S- AS. ii. Show that for the resulting matrix, S- AS = -1 2 [ A] 1 b 1 and 5 by determining and then simplifying its characteristic equation. 2 the 2 x 2 matrix A has eigenvalues iii. Find the eigenvector from A corresponding with = 1 and then construct an orthogonal 2 x 2 matrix Q where the first column is based on your eigenvector. Hence construct the matrix 1 00 [ 0 Q iv. Calculate P = SR. Show that P is an orthogonal matrix and verify that P-AP is upper triangular. 5 marks