There are just three assets with rate of return r₁, r₂, and r₃, respectively. The covariance matrix and the expected rates of return are as follows: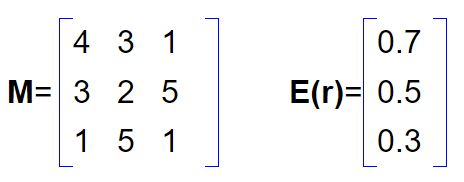

Consider the portfolio of the three assets. (a) Suppose that the weight vector of portfolio A is W_A = (0.5, 0.2, 0.3), find its expected rate of return and standard deviation of the rate of return.

(b) Find the weight vector of the minimum-variance portfolio B.

(c) Let r_A and r_B denote the random rate of return of portfolio A and portfolio B, respectively. Find the covariance between r_A and r_B, and then determine if r_A and r_B are correlated or not. (Positively correlated, Negatively correlated and Uncorrelated.)

(d) Can we find a portfolio whose rate of return is uncorrelated with that of portfolio A? If yes, find the portfolio. If no, explain why.

4 3 1 M= 3 2 5 0.7 E(r)= 0.5 0.3 15 1 4 3 1 M= 3 2 5 0.7 E(r)= 0.5 0.3 15 1