These two questions are from the book 'Stochastic Calculus for Finance 1' by Steven Shreve. Please solve these two questions from the book in detail - mention all the steps very clearly and also provide explanation as it helps in understanding. Please write the solution in very neat handwriting and try to not post a blur picture. Thank you in advance!

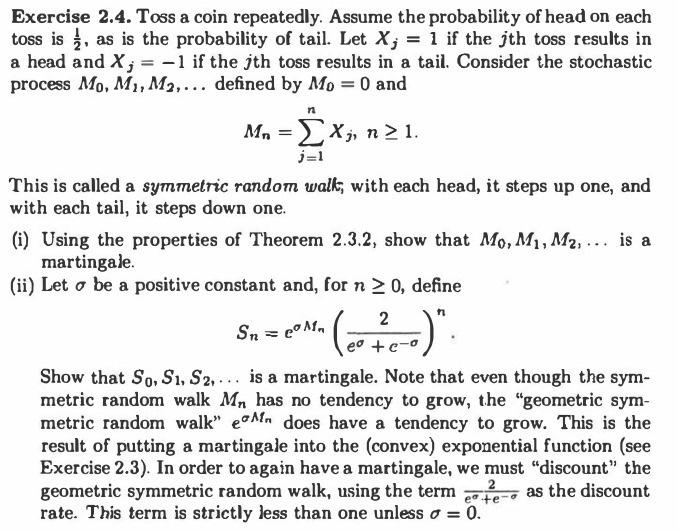
Exercise 1.5. In Example 1.2.4, we considered an agent who sold the look- back option for Vo = 1.376 and bought 40 = 0.1733 shares of stock at time zero. At time one, if the stock goes up, she has a portfolio valued at Vi(H) = 2.24. Assume that she now takes a position of A (H) = Vz(HH)-V2(HT) S(HH)-S2(HT) in the stock. Show that, at time two, if the stock goes up again, she will have a port- folio valued at V2(HH) = 3.20, whereas if the stock goes down, her portfolio will be worth V2(HT) = 2.40. Finally, under the assumption that the stock goes up in the first period and down in the second period, assume the agent takes a position of Az(HT) = S(HTH)-S3(HTT) in the stock. Show that, at time three, if the stock goes up in the third period, she will have a portfolio valued at V3(HTH) = 0, whereas if the stock goes down, her portfolio will be worth V3(HTT) = 6. In other words, she has hedged her short position in the option. Exercise 2.4. Toss a coin repeatedly. Assume the probability of head on each toss is , as is the probability of tail. Let X; = 1 if the jth toss results in a head and X; = -1 if the jth toss results in a tail. Consider the stochastic process Mo, M2,M3,... defined by Mo = 0 and Mn = xj, n21. j=1 This is called a symmetric random wall, with each head, it steps up one, and with each tail, it steps down one. (i) Using the properties of Theorem 2.3.2, show that Mo,M1, M2, ... is a martingale. (ii) Let o be a positive constant and, for n > 0, define 2 eo + c Show that So, S1, S2.... is a martingale. Note that even though the sym- metric random walk M, has no tendency to grow, the "geometric sym- metric random walk" oln does have a tendency to grow. This is the result of putting a martingale into the convex) exponential function (see Exercise 2.3). In order to again have a martingale, we must "discount" the geometric symmetric random walk, using the term cate, as the discount rate. This term is strictly less than one unless o = 0. Sn = eoM Exercise 1.5. In Example 1.2.4, we considered an agent who sold the look- back option for Vo = 1.376 and bought 40 = 0.1733 shares of stock at time zero. At time one, if the stock goes up, she has a portfolio valued at Vi(H) = 2.24. Assume that she now takes a position of A (H) = Vz(HH)-V2(HT) S(HH)-S2(HT) in the stock. Show that, at time two, if the stock goes up again, she will have a port- folio valued at V2(HH) = 3.20, whereas if the stock goes down, her portfolio will be worth V2(HT) = 2.40. Finally, under the assumption that the stock goes up in the first period and down in the second period, assume the agent takes a position of Az(HT) = S(HTH)-S3(HTT) in the stock. Show that, at time three, if the stock goes up in the third period, she will have a portfolio valued at V3(HTH) = 0, whereas if the stock goes down, her portfolio will be worth V3(HTT) = 6. In other words, she has hedged her short position in the option. Exercise 2.4. Toss a coin repeatedly. Assume the probability of head on each toss is , as is the probability of tail. Let X; = 1 if the jth toss results in a head and X; = -1 if the jth toss results in a tail. Consider the stochastic process Mo, M2,M3,... defined by Mo = 0 and Mn = xj, n21. j=1 This is called a symmetric random wall, with each head, it steps up one, and with each tail, it steps down one. (i) Using the properties of Theorem 2.3.2, show that Mo,M1, M2, ... is a martingale. (ii) Let o be a positive constant and, for n > 0, define 2 eo + c Show that So, S1, S2.... is a martingale. Note that even though the sym- metric random walk M, has no tendency to grow, the "geometric sym- metric random walk" oln does have a tendency to grow. This is the result of putting a martingale into the convex) exponential function (see Exercise 2.3). In order to again have a martingale, we must "discount" the geometric symmetric random walk, using the term cate, as the discount rate. This term is strictly less than one unless o = 0. Sn = eoM