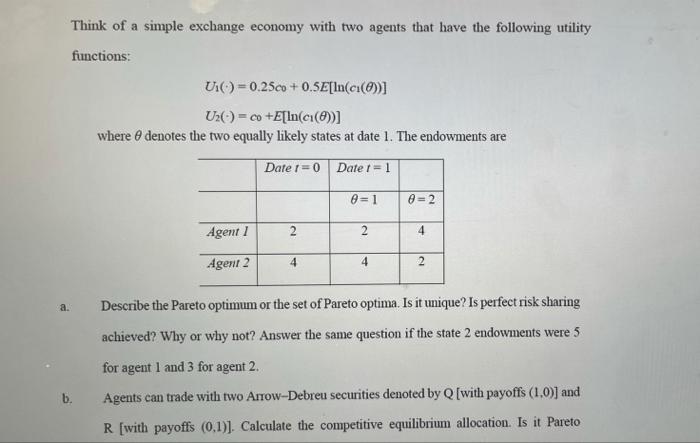
Think of a simple exchange economy with two agents that have the following utility functions: UIO0.25co + 0.5E[In(c(0)] U20- = co +E[ln(c(0)] where e denotes the two equally likely states at date 1. The endowments are Date=0 Date = 1 =1 0= 2 Agent 1 2 2 4 Agent 2 4 4 2 a. Describe the Pareto optimum or the set of Pareto optima. Is it unique? Is perfect risk sharing achieved? Why or why not? Answer the same question if the state 2 endowments were 5 for agent 1 and 3 for agent 2. Agents can trade with two Arrow-Debreu securities denoted by Q [with payoffs (1.0)) and R [with payoffs (0,1)]. Calculate the competitive equilibrium allocation. Is it Pareto b. Agent / 2 2 4 Agent 2 4 4 al 2 a b. Describe the Pareto optimum or the set of Pareto optima. Is it unique? Is perfect risk sharing achieved? Why or why not? Answer the same question if the state 2 endowments were 5 for agent 1 and 3 for agent 2. Agents can trade with two Arrow-Debreu securities denoted by Q [with payoffs (1.0)) and R (with payoffs (0,1)]. Calculate the competitive equilibrium allocation. Is it Pareto optimal? Discuss intuitively its characteristics (the determinants of the prices of the two securities and the post-trade allocation). Suppose that both securities can be traded at the beginning and there are no short-selling constraints. A firm has zero cost of introducing one unit of either security, and it wants to maximize its profits. Which security should it introduce? For which agent is this introduction more valuable? Is the outcome Pareto optimal? Why or why not? Think of a simple exchange economy with two agents that have the following utility functions: UIO0.25co + 0.5E[In(c(0)] U20- = co +E[ln(c(0)] where e denotes the two equally likely states at date 1. The endowments are Date=0 Date = 1 =1 0= 2 Agent 1 2 2 4 Agent 2 4 4 2 a. Describe the Pareto optimum or the set of Pareto optima. Is it unique? Is perfect risk sharing achieved? Why or why not? Answer the same question if the state 2 endowments were 5 for agent 1 and 3 for agent 2. Agents can trade with two Arrow-Debreu securities denoted by Q [with payoffs (1.0)) and R [with payoffs (0,1)]. Calculate the competitive equilibrium allocation. Is it Pareto b. Agent / 2 2 4 Agent 2 4 4 al 2 a b. Describe the Pareto optimum or the set of Pareto optima. Is it unique? Is perfect risk sharing achieved? Why or why not? Answer the same question if the state 2 endowments were 5 for agent 1 and 3 for agent 2. Agents can trade with two Arrow-Debreu securities denoted by Q [with payoffs (1.0)) and R (with payoffs (0,1)]. Calculate the competitive equilibrium allocation. Is it Pareto optimal? Discuss intuitively its characteristics (the determinants of the prices of the two securities and the post-trade allocation). Suppose that both securities can be traded at the beginning and there are no short-selling constraints. A firm has zero cost of introducing one unit of either security, and it wants to maximize its profits. Which security should it introduce? For which agent is this introduction more valuable? Is the outcome Pareto optimal? Why or why not