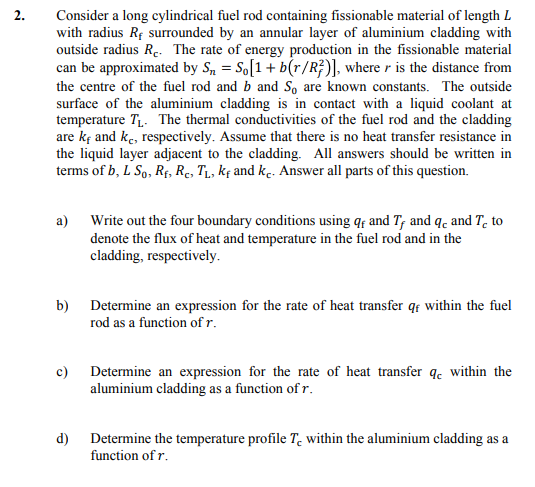
This information is also given for help

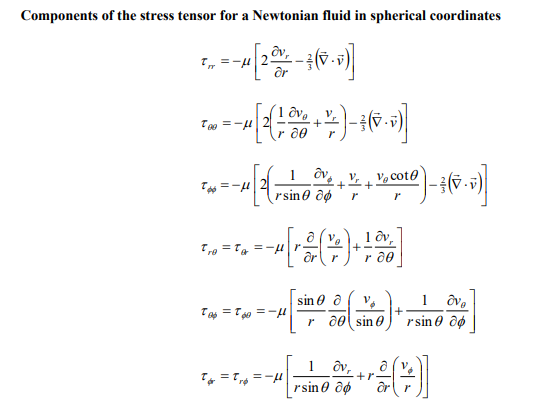
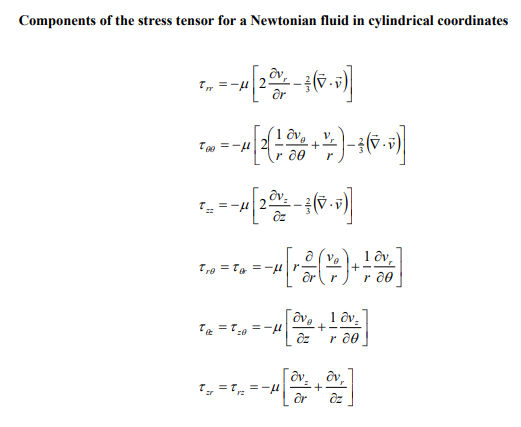
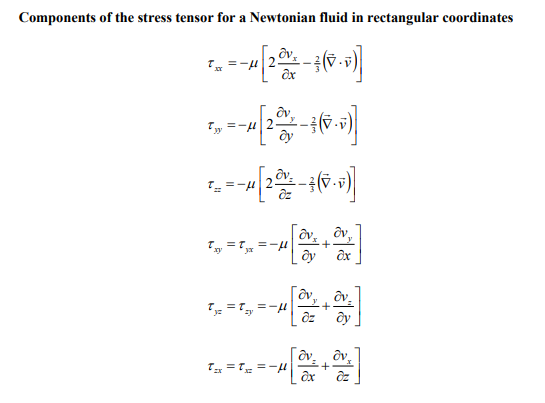
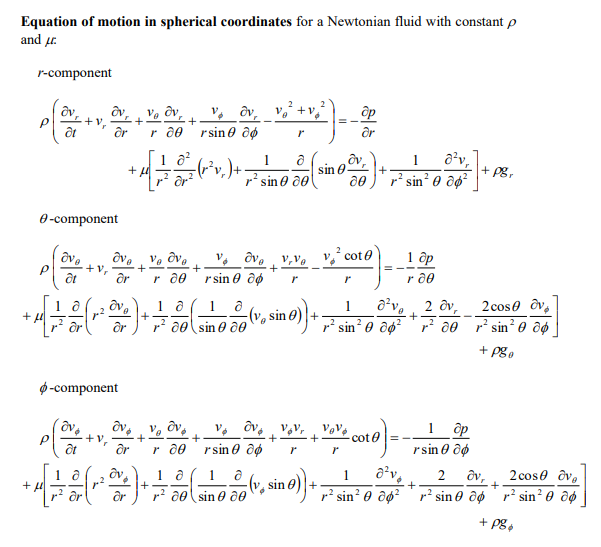
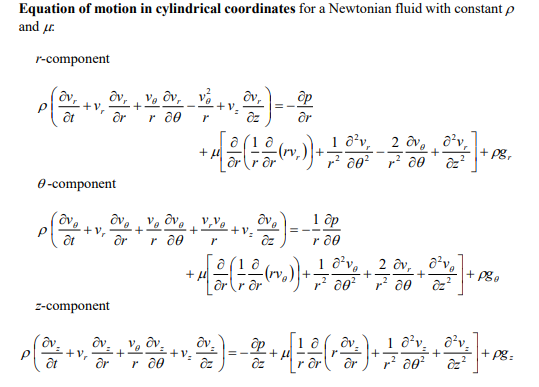
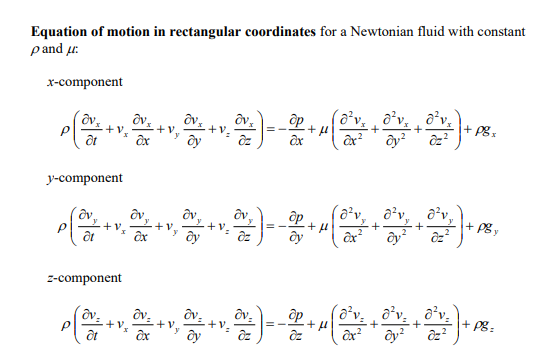Consider a long cylindrical fuel rod containing fissionable material of length L with radius Rf surrounded by an annular layer of aluminium cladding with outside radius Rc. The rate of energy production in the fissionable material can be approximated by Sn=S0[1+b(r/Rf2)], where r is the distance from the centre of the fuel rod and b and S0 are known constants. The outside surface of the aluminium cladding is in contact with a liquid coolant at temperature TL. The thermal conductivities of the fuel rod and the cladding are kf and kc, respectively. Assume that there is no heat transfer resistance in the liquid layer adjacent to the cladding. All answers should be written in terms of b,LS0,Rf,Rc,TL,kf and kc. Answer all parts of this question. a) Write out the four boundary conditions using qf and Tf and qc and Tc to denote the flux of heat and temperature in the fuel rod and in the cladding, respectively. b) Determine an expression for the rate of heat transfer qf within the fuel rod as a function of r. c) Determine an expression for the rate of heat transfer qc within the aluminium cladding as a function of r. d) Determine the temperature profile Tc within the aluminium cladding as a function of r. e) Determine the temperature profile Tf within the fuel rod as a function of r. f) Calculate the rate of energy generation by the fission reaction. g) Calculate the maximum temperature in the fuel rod. h) Calculate the average temperature in the cladding. Components of the stress tensor for a Newtonian fluid in spherical coordinates rr=[2rvr32(v)]=[2(r1v+rvr)32(v)]=[2(rsin1v+rvr+rvcot)32(v)]r==[rr(rv)+r1vr]==[rsin(sinv)+rsin1v]=r=[rsin1vr+rr(rv)] Components of the stress tensor for a Newtonian fluid in cylindrical coordinates rr=[2rvr32(v)]=[2(r1v+rvr)32(v)]zz=[2zvz32(v)]r=r=[rr(rv)+r1vr]z=z=[zv+r1vz]zr=rz=[rvz+zvr] Components of the stress tensor for a Newtonian fluid in rectangular coordinates xx=[2xvx32(v)]yy=[2yvy32(v)]zz=[2zvz32(v)]xy=yx=[yvx+xvy]yz=zy=[zvy+yvz]zx=xz=[xvz+zvx] Equation of motion in spherical coordinates for a Newtonian fluid with constant and . r-component (tvr+vrrvr+rvvr+rsinvvrrv2+v2)=rp+[r21r22(r2vr)+r2sin1(sinvr)+r2sin2122vr]+gr -component (tv+vrrv+rvv+rsinvv+rvrvrv2cot)=r1p+[r21r(r2rv)+r21(sin1(vsin))+r2sin2122v+r22vrr2sin22cosv]+g -component (tv+vrrv+rvv+rsinvv+rvvr+rvvcot)=rsin1p+[r21r(r2rv)+r21(sin1(vsin))+r2sin2122v+r2sin2vr+r2sin22cosv]+g Equation of motion in cylindrical coordinates for a Newtonian fluid with constant and . r-component (tvr+vrrvr+rvvrrv2+vzzvr)=rp+[r(r1r(rvr))+r2122vrr22v+z22vr]+gr -component (tv+vrrv+rvv+rvrv+vzzv)=r1p+[r(r1r(rv))+r2122v+r22vr+z22v]+gz-component(tvz+vrrvz+rvvz+vzzvz)=zp+[r1r(rrvz)+r2122vz+z22vz]+gz Equation of motion in rectangular coordinates for a Newtonian fluid with constant and : x-component (tvx+vxxvx+vyyvx+vzzvx)=xp+(x22vx+y22vx+z22vx)+gx y-component (tvy+vxxvy+vyyvy+vzzvy)=yp+(x22vy+y22vy+z22vy)+gy z-component (tvz+vxxvz+vyyvz+vzzvz)=zp+(x22vz+y22vz+z22vz)+gz