This is Matlab question of Linear Algebra, and it should be solved by using Matlab. Need the code and script of the two question.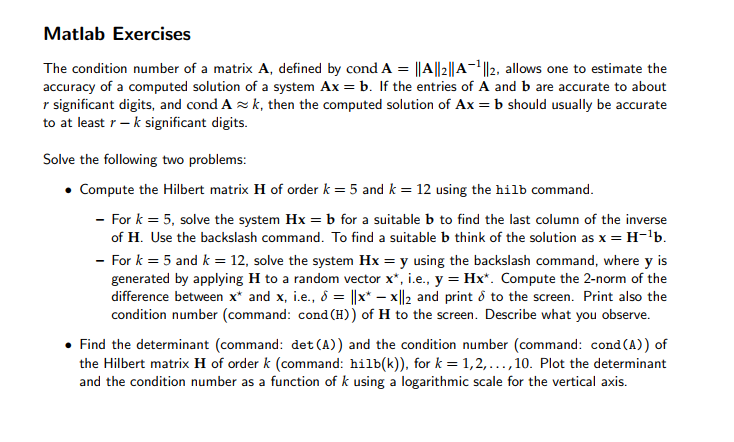

Matlab Exercises The condition number of a matrix A, defined by cond A All2A-12, allows one to estimate the accuracy of a computed solution of a system Ax = b. If the entries of A and b are accurate to about r significant digits, and cond A ~ k, then the computed solution of Ax = b should usually be accurate to at least r-k significant digits. Solve the following two problems: Compute the Hilbert matrix H of order k- 5 and k 12 using the hilb command. -For k = 5, solve the system Hx b for a suitable b to find the last column of the inverse of H. Use the backslash command. To find a suitable b think of the solution as x - H-1b. - For k- 5 and k 12, solve the system Hx - y using the backslash command, where y is generated by applying H to a random vector x*, ie., y-Hx". Compute the 2-norm of the difference between x* and x, i.e., ? Hlx*-x112 and print ? to the screen. Print also the condition number (command: cond CH)) of H to the screen. Describe what you observe. Find the determinant (command: det (A)) and the condition number (command: cond (A)) of the Hilbert matrix H of order k (command: hilb(k)), for k- 1,2,...,10. Plot the determinant and the condition number as a function of k using a logarithmic scale for the vertical axis. Matlab Exercises The condition number of a matrix A, defined by cond A All2A-12, allows one to estimate the accuracy of a computed solution of a system Ax = b. If the entries of A and b are accurate to about r significant digits, and cond A ~ k, then the computed solution of Ax = b should usually be accurate to at least r-k significant digits. Solve the following two problems: Compute the Hilbert matrix H of order k- 5 and k 12 using the hilb command. -For k = 5, solve the system Hx b for a suitable b to find the last column of the inverse of H. Use the backslash command. To find a suitable b think of the solution as x - H-1b. - For k- 5 and k 12, solve the system Hx - y using the backslash command, where y is generated by applying H to a random vector x*, ie., y-Hx". Compute the 2-norm of the difference between x* and x, i.e., ? Hlx*-x112 and print ? to the screen. Print also the condition number (command: cond CH)) of H to the screen. Describe what you observe. Find the determinant (command: det (A)) and the condition number (command: cond (A)) of the Hilbert matrix H of order k (command: hilb(k)), for k- 1,2,...,10. Plot the determinant and the condition number as a function of k using a logarithmic scale for the vertical axis