this is one , drop down boxes have options, please show how you got your answers

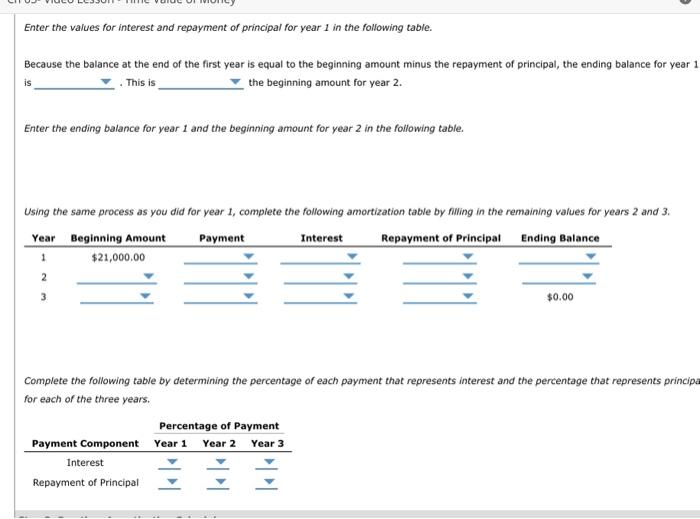
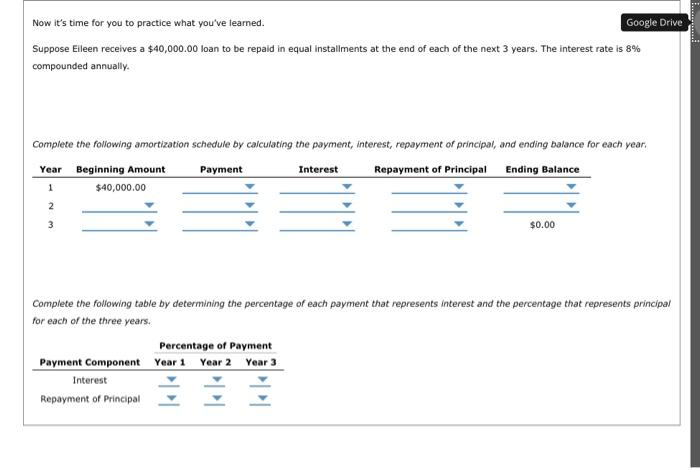
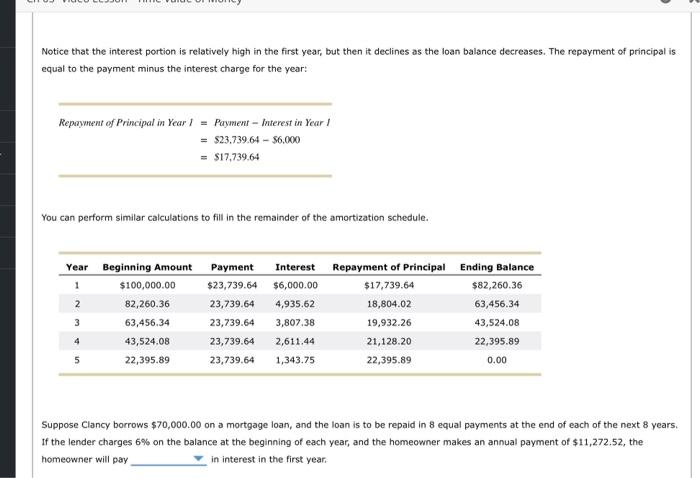
Suppose Clancy receives a $21,000.00 loan to be repaid in equal installments at the end of each of the next 3 years. The interest rate is 4% compounded annually. Use the formula for the present value of an ordinary annuity to find this payment amount: PVANPMT=PMT1(11+1)=PVAN(11+n1)1 In this case, PVAN equals , 1 equals , and N equals Using the formula for the present value of an ordinary annuity, the annual payment amount for this loan is Because this payment is fixed over time, enter this annual payment amount in the "Payment" column of the following table for all three years. Each payment consists of two parts-interest and repayment of principal. You can calculate the interest in year 1 by multiplying the loan balance at the beginning of the year (PVAN) by the interest rate (I). The repayment of principal is equal to the payment (PMT) minus the interest charge for the year: The interest paid in year 1 is Enter the values for interest and repayment of principal for year 1 in the following table. Enter the values for interest and repayment of principal for year 1 in the following table. Because the balance at the end of the first year is equal to the beginning amount minus the repayment of principal, the ending balance for year is . This is the beginning amount for year 2 . Enter the ending balance for year 1 and the beginning amount for year 2 in the following table. Using the same process as you did for year 1 , complete the following amortization table by filing in the remaining values for years 2 and 3 . Complete the following table by determining the percentage of each payment that represents interest and the percentage that represents princip. for each of the three years. Now it's time for you to practice what you've learned. Suppose Eileen receives a $40,000.00 loan to be repaid in equal installments at the end of each of the next 3 years. The interest rate is 8% compounded annually. Complete the following amortization schedule by calculating the payment, interest, repayment of principal, and ending balance for each year. Complete the following table by determining the percentage of each payment that represents interest and the percentage that represents principal for each of the three years. Notice that the interest portion is relatively high in the first year, but then it declines as the loan balance decreases. The repayment of principal is equal to the payment minus the interest charge for the year: You can perform similar calculations to fill in the remainder of the amortization schedule. Suppose Clancy borrows $70,000.00 on a mortgage loan, and the loan is to be repaid in 8 equal payments at the end of each of the next 8 years, If the lender charges 6% on the balance at the beginning of each year, and the homeowner makes an annual payment of $11,272.52, the homeowner will pay in interest in the first year