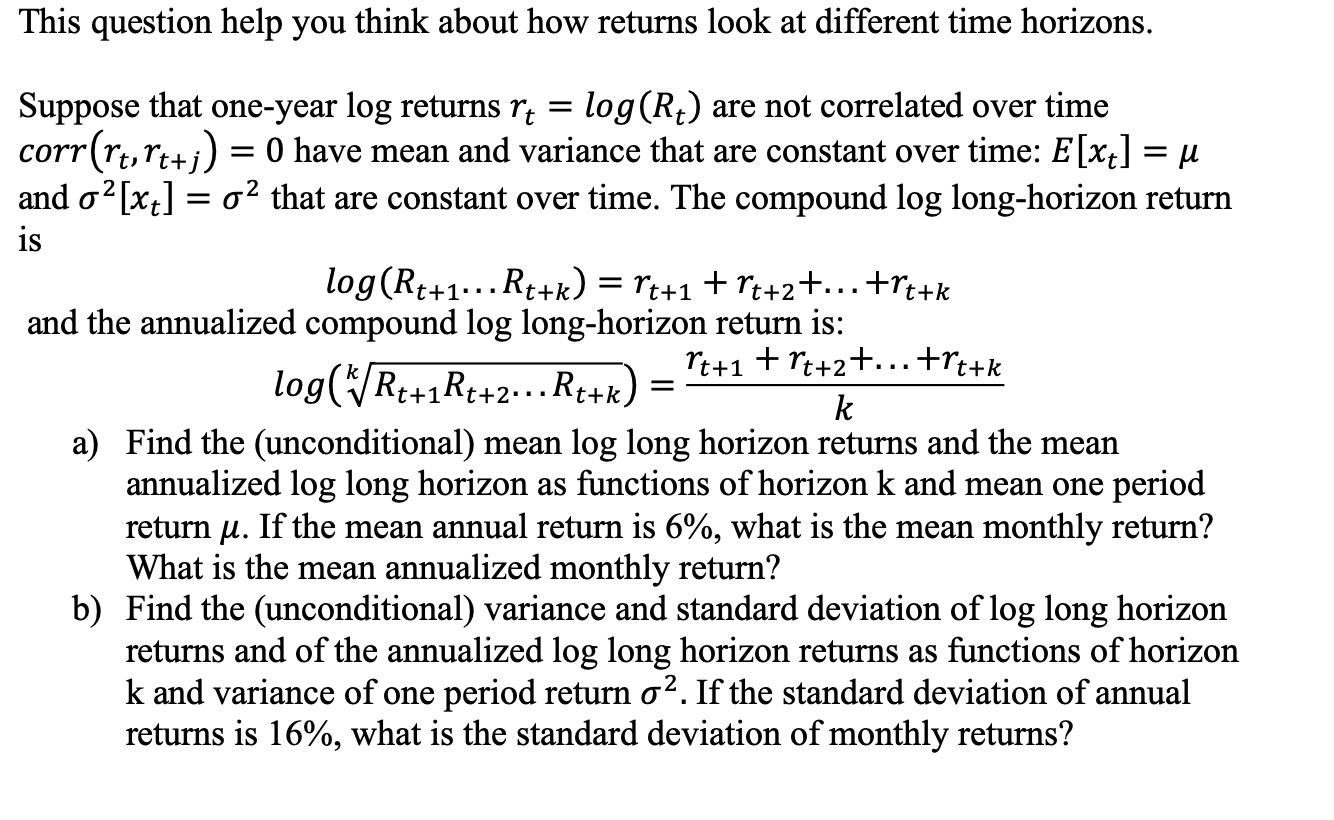
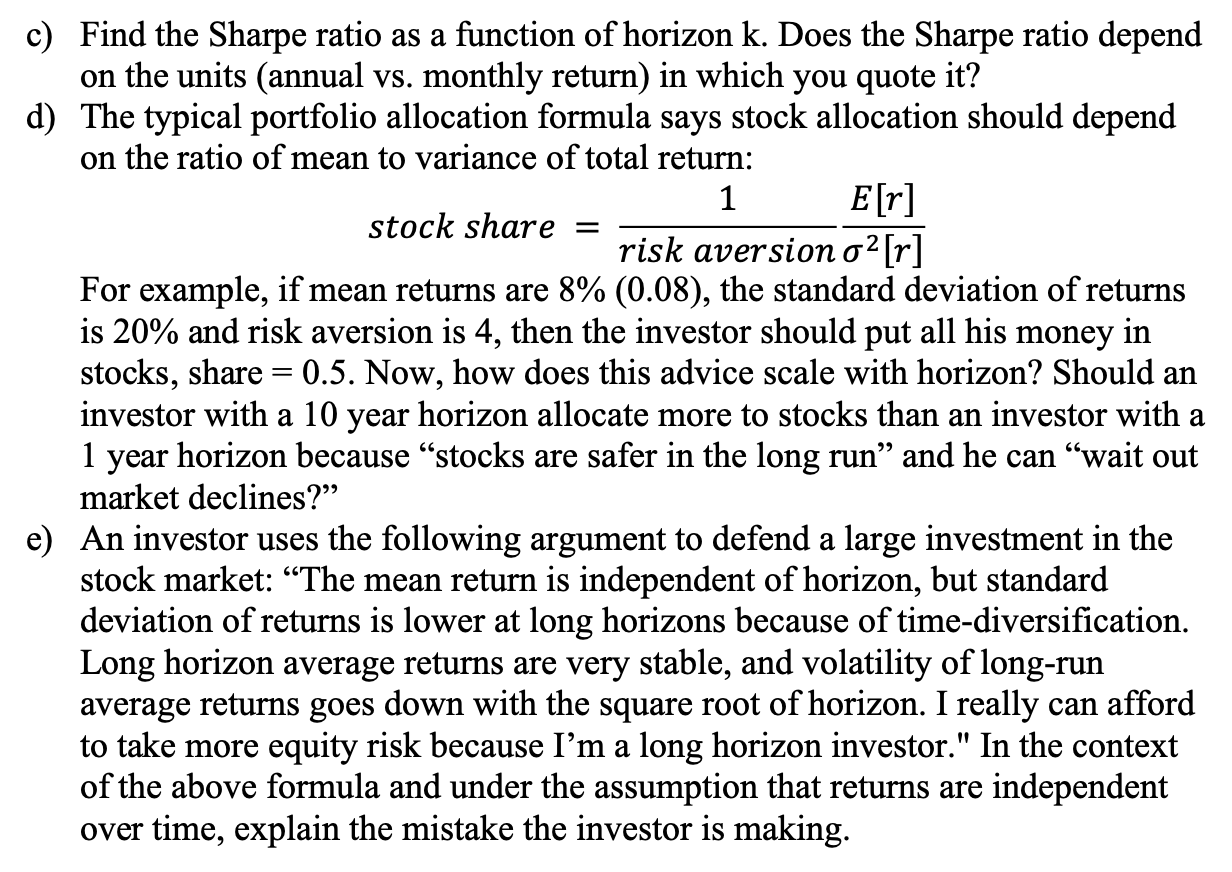This question help you think about how returns look at different time horizons. Suppose that one-year log returns rt=log(Rt) are not correlated over time corr(rt,rt+j)=0 have mean and variance that are constant over time: E[xt]= and 2[xt]=2 that are constant over time. The compound log long-horizon return is log(Rt+1Rt+k)=rt+1+rt+2++rt+k and the annualized compound log long-horizon return is: log(kRt+1Rt+2Rt+k)=krt+1+rt+2++rt+k a) Find the (unconditional) mean log long horizon returns and the mean annualized log long horizon as functions of horizon k and mean one period return . If the mean annual return is 6%, what is the mean monthly return? What is the mean annualized monthly return? b) Find the (unconditional) variance and standard deviation of log long horizon returns and of the annualized log long horizon returns as functions of horizon k and variance of one period return 2. If the standard deviation of annual returns is 16%, what is the standard deviation of monthly returns? c) Find the Sharpe ratio as a function of horizon k. Does the Sharpe ratio depend on the units (annual vs. monthly return) in which you quote it? d) The typical portfolio allocation formula says stock allocation should depend on the ratio of mean to variance of total return: stockshare=riskaversion12[r]E[r] For example, if mean returns are 8%(0.08), the standard deviation of returns is 20% and risk aversion is 4 , then the investor should put all his money in stocks, share =0.5. Now, how does this advice scale with horizon? Should an investor with a 10 year horizon allocate more to stocks than an investor with a 1 year horizon because "stocks are safer in the long run" and he can "wait out market declines?" e) An investor uses the following argument to defend a large investment in the stock market: "The mean return is independent of horizon, but standard deviation of returns is lower at long horizons because of time-diversification. Long horizon average returns are very stable, and volatility of long-run average returns goes down with the square root of horizon. I really can afford to take more equity risk because I'm a long horizon investor." In the context of the above formula and under the assumption that returns are independent over time, explain the mistake the investor is making