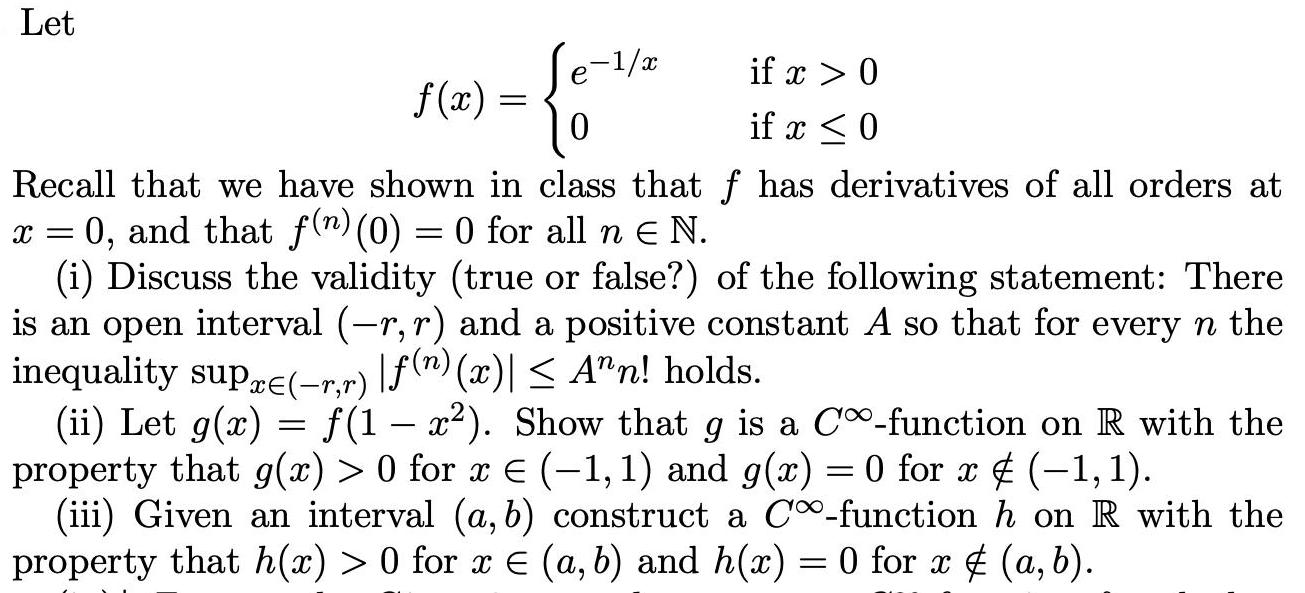
Let ,-1/x if x > 0 e f(x) = 0. if x < 0 Recall that we have shown in class that f has derivatives of all orders at x = 0, and that f(n) (0) = 0 for all n E N. (i) Discuss the validity (true or false?) of the following statement: There is an open interval (-r, r) and a positive constant A so that for every n the inequality supxE(-r,r) (ii) Let g(x) = f(1 x). Show that g is a Co0-function on R with the property that g(x) > 0 for x E (-1,1) and g(x) = 0 for x (-1,1). (iii) Given an interval (a, b) construct a Co-function h on R with the property that h(x) > 0 for x E (a, b) and h(x) = 0 for x (a, b). |f(") (x)| < A"n! holds. %3D