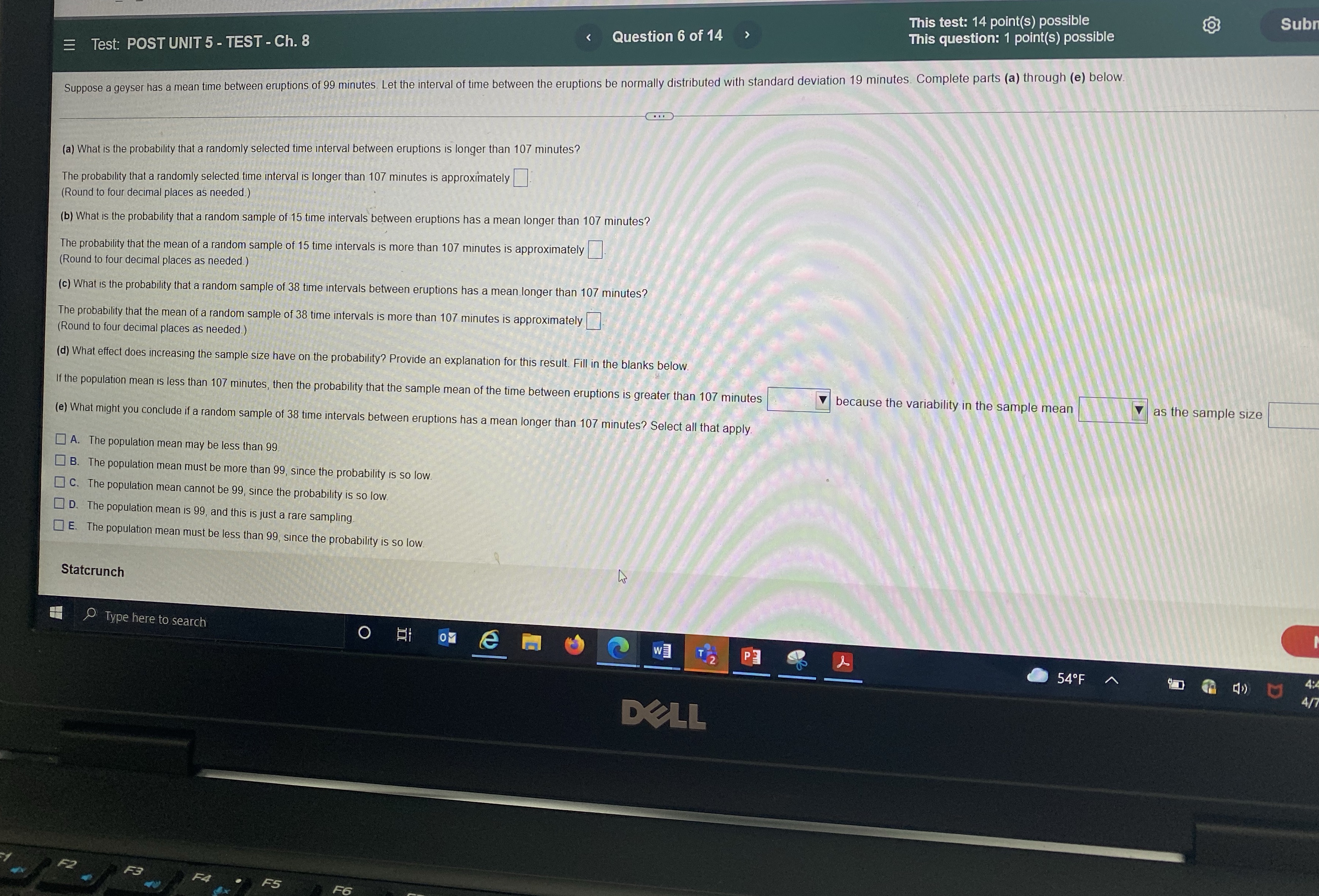
This test: 14 point(s) possible Sub Question 6 of 14 > This question: 1 point(s) possible E Test: POST UNIT 5 - TEST - Ch. 8 Suppose a geyser has a mean time between eruptions of 99 minutes. Let the interval of time between the eruptions be normally distributed with standard deviation 19 minutes. Complete parts (a) through (e) below. (a) What is the probability that a randomly selected time interval between eruptions is longer than 107 minutes? The probability that a randomly selected time interval is longer than 107 minutes is approximately (Round to four decimal places as needed.) (b) What is the probability that a random sample of 15 time intervals between eruptions has a mean longer than 107 minutes? The probability that the mean of a random sample of 15 time intervals is more than 107 minutes is approximately (Round to four decimal places as needed.) (c) What is the probability that a random sample of 38 time intervals between eruptions has a mean longer than 107 minutes? The probability that the mean of a random sample of 38 time intervals is more than 107 minutes is approximately (Round to four decimal places as needed.) d) What effect does increasing the sample size have on the probability? Provide an explanation for this result. Fill in the blanks below. If the population mean is less than 107 minutes, then the probability that the sample mean of the time between eruptions is greater than 107 minutes because the variability in the sample mean as the sample size (e) What might you conclude if a random sample of 38 time intervals between eruptions has a mean longer than 107 minutes? Select all that apply. A. The population mean may be less than 99. B. The population mean must be more than 99, since the probability is so low. C. The population mean cannot be 99, since the probability is so low. D. The population mean is 99, and this is just a rare sampling. E. The population mean must be less than 99, since the probability is so low. Statcrunch Type here to search O WE T 2 Ed 54OF 4/ DELL F5