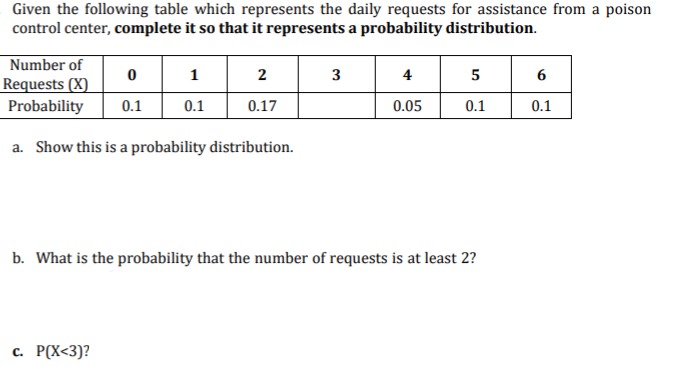
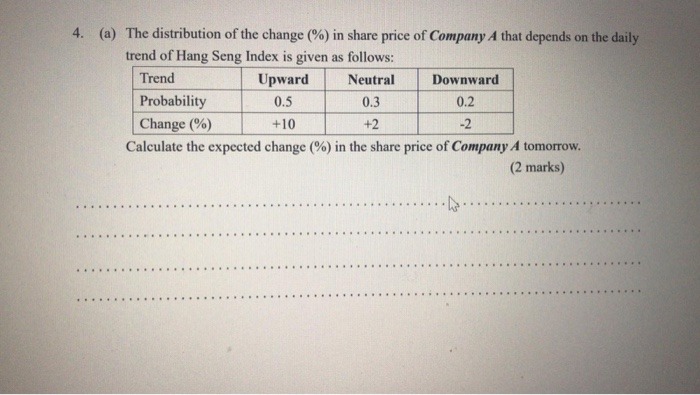
Question
Three prisoners, A, B, and C, are held in separate cells. Two are to be executed. The warder knows specifically who is to be executed,
Three prisoners, A, B, and C, are held in separate cells. Two are to be executed. The warder knows
specifically who is to be executed, and who is to be freed, whereas the prisoners know only that two
are to be executed. Prisoner A reasons as follows: my probability of being freed is clearly 1
3
until I
receive further information. However, it is clear that at least one of B and C will be executed, so I
will ask the warder to name one prisoner other than myself who is to be executed. Once I know which
of B and C is to be executed, either I will go free or the other, unnamed, prisoner will go free, with
equal probability. Hence, by asking the name of another prisoner to be executed, I raise my chances
of survival from 1
3
to 1
2
. Investigate A's reasoning. [Hint: find the conditional probability that A is
freed, given that the warder names B to be executed.]
Given the accompanying information for a fanciful hero universe:
Flying Telepathy Super Strength
Female 97 92 77
Male 24 64 3
Dissemination of Super Power by Gender
What is the observational likelihood that a haphazardly chosen hero will have clairvoyance?
Answer in decimal structure. Round to 3 decimal places on a case by case basis
An overview asked 816 school green beans whether they had been to a film or eaten in a caf during the previous week. The accompanying data was acquired: 391 green beans had been to neither a film nor an eatery, and 266 had been to a film. In the event that 92 of the individuals who had been to a film had not been to a caf, what number of the reviewed green beans had been to the accompanying?
(a) both a film and a caf
(b) a film or a caf
(c) a caf
(d) a caf however not a film
A rescue vehicle goes to and fro, at a steady explicit speed v, along a street of length L. We may show the area of the emergency vehicle at any second on schedule to be consistently circulated over the span (0, L). Additionally at any second on schedule, a mishap (not including the emergency vehicle itself) happens at a point consistently disseminated out and about; that is, the mishaps distance from one of the fixed stopping points is likewise consistently conveyed over the stretch (0, L). Expect the area of the mishap and the area of the rescue vehicle are free.
Assuming the emergency vehicle is fit for guaranteed U-turns, register the CDF and PDF of the ambulances head out time T to the area of the mishap.
Alex Mason has a wide-bending, uphill carport prompting
~28~
his carport. When there is a weighty snow, Alex enlists a nearby
woodworker, who digging tools snow as an afterthought in the colder time of year, to
scoop his carport. The snow shoveler charges $30 to
scoop the carport. Following is a likelihood conveyance
of the quantity of hefty snows each winter.Alex is thinking about the acquisition of another self-pushed
snowblower for $575 that would permit him, his better half, or his
kids to clear the carport after a snow. Talk about what
you believe Alex's choice ought to be and why.
Weighty Snows Probability
1 1.12
2 0.19
3 0.24
4 0.22
5 0.13
6 0.08
7 0.02


Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


