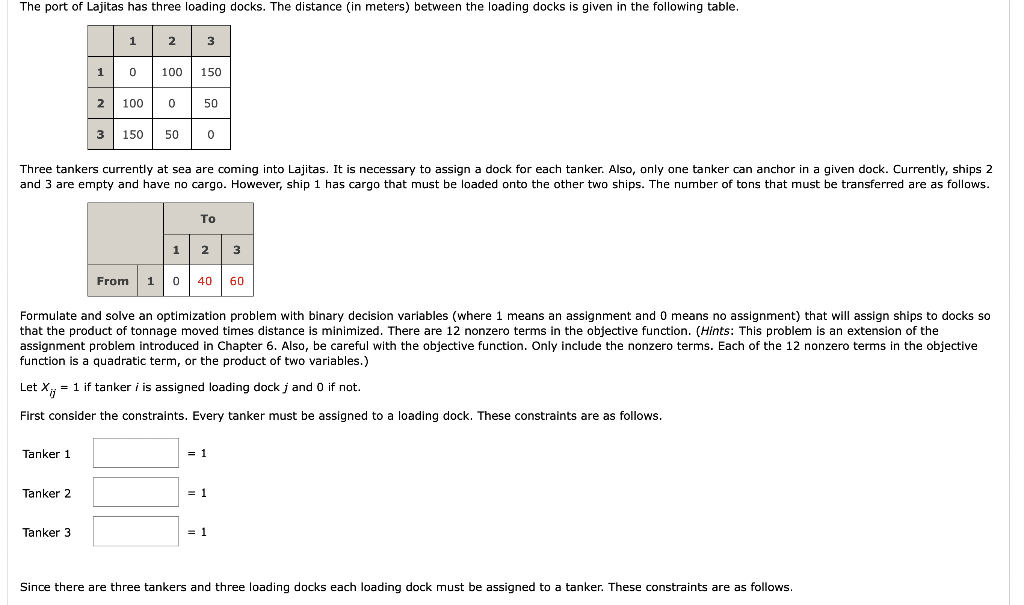

Three tankers currently at sea are coming into Lajitas. It is necessary to assign a dock for each tanker. Also, only one tanker can anchor in a given dock. Currently, ships 2 and 3 are empty and have no cargo. However, ship 1 has cargo that must be loaded onto the other two ships. The number of tons that must be transferred are as follows. Formulate and solve an optimization problem with binary decision variables (where 1 means an assignment and 0 means no assignment) that will assign ships to docks so that the product of tonnage moved times distance is minimized. There are 12 nonzero terms in the objective function. (Hints: This problem is an extension of the assignment problem introduced in Chapter 6 . Also, be careful with the objective function. Only include the nonzero terms. Each of the 12 nonzero terms in the objective function is a quadratic term, or the product of two variables.) Let Xij=1 if tanker i is assigned loading dock j and 0 if not. First consider the constraints. Every tanker must be assigned to a loading dock. These constraints are as follows. Tanker 1=1 Tanker 2=1 Tanker 3=1 Dock 1 =1 Dock 2=1 Dock 3=1 The complete objective function is found by considering the result of assigning tankers to docks and the product of distance and Complete the solution to the model. Let Xij=1 if tanker i is assigned loading dock j and 0 if not. x11= x12= x13= x21= x22= x23= x31= x32= x33= Objective value Three tankers currently at sea are coming into Lajitas. It is necessary to assign a dock for each tanker. Also, only one tanker can anchor in a given dock. Currently, ships 2 and 3 are empty and have no cargo. However, ship 1 has cargo that must be loaded onto the other two ships. The number of tons that must be transferred are as follows. Formulate and solve an optimization problem with binary decision variables (where 1 means an assignment and 0 means no assignment) that will assign ships to docks so that the product of tonnage moved times distance is minimized. There are 12 nonzero terms in the objective function. (Hints: This problem is an extension of the assignment problem introduced in Chapter 6 . Also, be careful with the objective function. Only include the nonzero terms. Each of the 12 nonzero terms in the objective function is a quadratic term, or the product of two variables.) Let Xij=1 if tanker i is assigned loading dock j and 0 if not. First consider the constraints. Every tanker must be assigned to a loading dock. These constraints are as follows. Tanker 1=1 Tanker 2=1 Tanker 3=1 Dock 1 =1 Dock 2=1 Dock 3=1 The complete objective function is found by considering the result of assigning tankers to docks and the product of distance and Complete the solution to the model. Let Xij=1 if tanker i is assigned loading dock j and 0 if not. x11= x12= x13= x21= x22= x23= x31= x32= x33= Objective value








