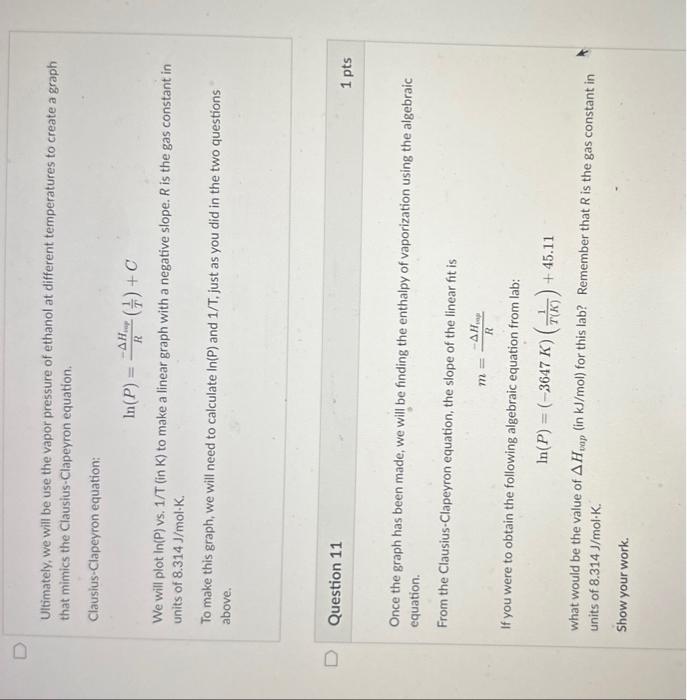
To first thing we need to do is determine the pressure of the atmospheric air in the flask at the higher temperature. When we are looking at the pressure of a gas at different temperatures, we can use the form of the ideal gas law when volumes and number of moles are held constant. Remember that whenever we use the gas law relationships, temperatures must be in K. T1P1=T2P2 We will use this equation to determine what the atmospheric air pressure is at different temperatures in a sealed flask. If atmospheric air in the flask at 21.2C has a pressure of 87.5kPa, what would be the pressure of the atmospheric air in the flask at 35.8C ? Show your work. Now that we have the pressure of the atmospheric air, we can subtract that from the total pressure measured at 35.8C to find the pressure of just the ethanol vapor. Using the pressure of the atmospheric air you found in question 7, and the measured pressure of 116.8kPa, determine the vapor pressure of the ethanol at 35.8C. Using the information from Question 8, calculate the natural log of the vapor pressure of ethanol. Calculate 1/T for the ethanol at 35.8C. You will need to record your values in decimal form, so make sure you have the appropriate number of significant figures. Edit View Insert Format Ultimately, we will be use the vapor pressure of ethanol at different temperatures to create a graph that mimics the Clausius-Clapeyron equation. Clausius-Clapeyron equation: ln(P)=RHiap(T1)+C We will plot ln(P) vs. 1/T (in K ) to make a linear graph with a negative slope. R is the gas constant in units of 8.314J/molK. To make this graph, we will need to calculate ln(P) and 1/T, just as you did in the two questions above. Question 11 1 pts Once the graph has been made, we will be finding the enthalpy of vaporization using the algebraic equation. From the Clausius-Clapeyron equation, the slope of the linear fit is m=RHiap If you were to obtain the following algebraic equation from lab: ln(P)=(3647K)(T(K)1)+45.11 what would be the value of Hvap (in kJ/mol ) for this lab? Remember that R is the gas constant in units of 8.314J/molK. Show your work