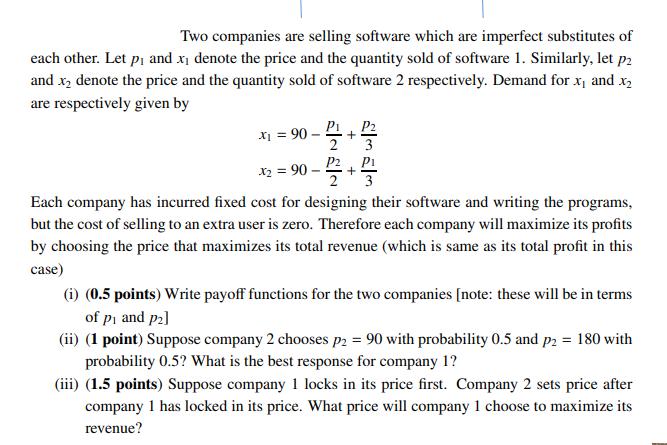
Two companies are selling software which are imperfect substitutes of each other. Let pi and x denote the price and the quantity sold of software 1. Similarly, let p2 and x2 denote the price and the quantity sold of software 2 respectively. Demand for x and x2 are respectively given by x = 90- Pi P2 + P2 P1 x290- + 2 Each company has incurred fixed cost for designing their software and writing the programs, but the cost of selling to an extra user is zero. Therefore each company will maximize its profits by choosing the price that maximizes its total revenue (which is same as its total profit in this case) (i) (0.5 points) Write payoff functions for the two companies [note: these will be in terms of p and p2] (ii) (1 point) Suppose company 2 chooses p2 = 90 with probability 0.5 and p2 = 180 with probability 0.5? What is the best response for company 1? (iii) (1.5 points) Suppose company 1 locks in its price first. Company 2 sets price after company 1 has locked in its price. What price will company 1 choose to maximize its revenue?