Two machines produce rivets for a factory job.

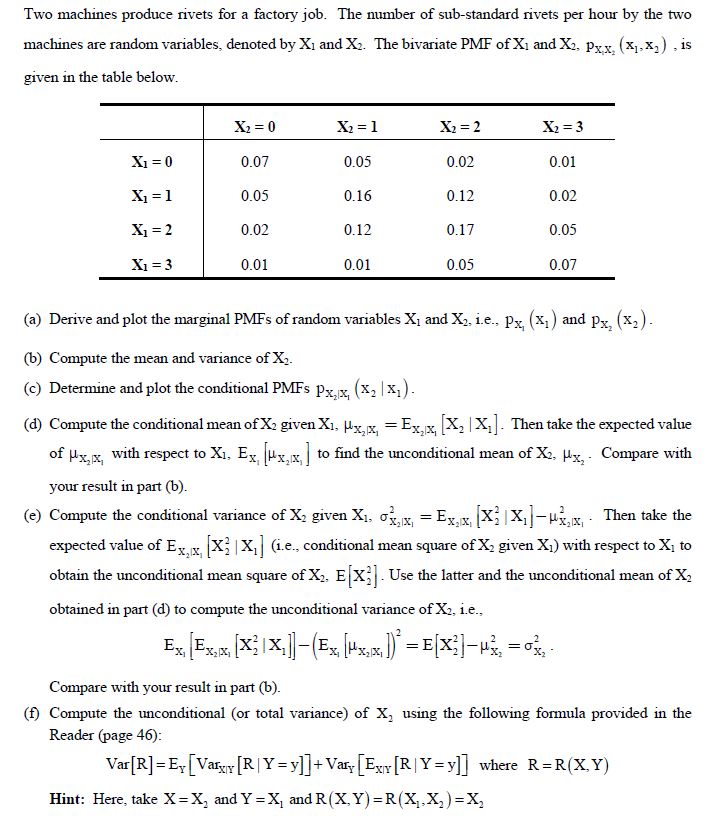
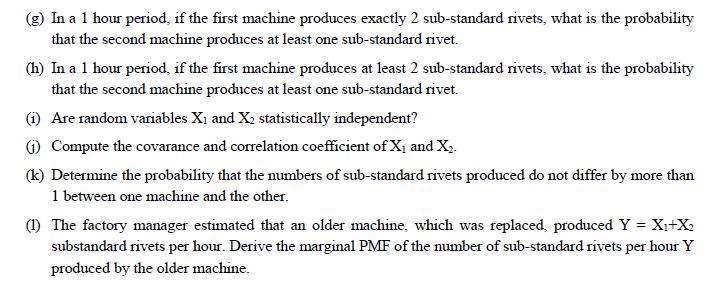
Two machines produce rivets for a factory job. The number of sub-standard rivets per hour by the two machines are random variables, denoted by Xi and X2. The bivariate PMF of Xi and X2. Px x, (X1.X, ) , is given in the table below. X2 = 0 X1 =1 X2 = 2 X2 =3 X1 = 0 0.07 0.05 0.02 0.01 X1 = 1 0.05 0.16 0.12 0.02 X1 = 2 0.02 0.12 0.17 0.05 X1 = 3 0.01 0.01 0.05 0.07 (a) Derive and plot the marginal PMFs of random variables X1 and X2, i.e., Px (x, ) and Px, (X2). b) Compute the mean and variance of X2. (c) Determine and plot the conditional PMFs Px, x, (X, | X] )- d) Compute the conditional mean of X2 given Xi, My, x, = Ex, x, [X, |X, ]. Then take the expected value of Ux, x, with respect to Xi. Ex, Hy, x, |to find the unconditional mean of X1. My, - Compare with your result in part (b). (e) Compute the conditional variance of X2 given X1, Ox, Ix, - Ex, x, (X} |X] ] -Hx, x, . Then take the expected value of Ex, x X; | X, (i.e., conditional mean square of X2 given X1) with respect to X] to obtain the unconditional mean square of X2, E X? . Use the latter and the unconditional mean of X2 obtained in part (d) to compute the unconditional variance of X2, i.e., Ex, Ex, x, [x; IX,] -(Ex, [ Mx x, ]) = =[X ]-MK =03 . Compare with your result in part (b). (f) Compute the unconditional (or total variance) of X, using the following formula provided in the Reader (page 46): Var [R] = Ey [Vary [R | Y =y]]+ Vary [Exy [R|Y =y]] where R=R(X, Y) Hint: Here, take X = X, and Y =X, and R(X, Y) =R(X X, ) =X,(g) In a 1 hour period, if the first machine produces exactly 2 sub-standard rivets, what is the probability that the second machine produces at least one sub-standard rivet. (h) In a 1 hour period, if the first machine produces at least 2 sub-standard rivets, what is the probability that the second machine produces at least one sub-standard rivet. (i) Are random variables X1 and X2 statistically independent? (j) Compute the covarance and correlation coefficient of X, and Xz. (k) Determine the probability that the numbers of sub-standard rivets produced do not differ by more than 1 between one machine and the other. (1) The factory manager estimated that an older machine, which was replaced, produced Y = Xi+X2 substandard rivets per hour. Derive the marginal PMF of the number of sub-standard rivets per hour Y produced by the older machine