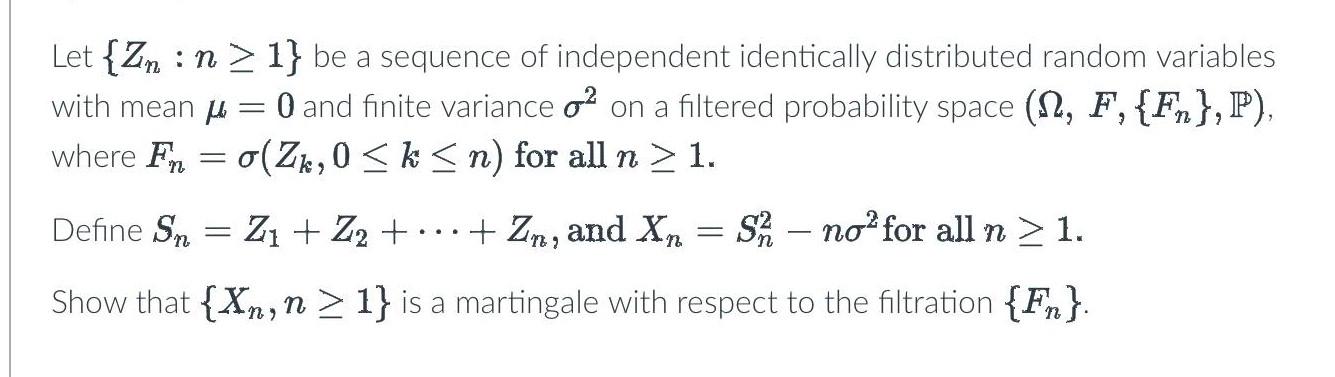
Urgently! Need it right now!

Let {Zn:n1} be a sequence of independent identically distributed random variables with mean =0 and finite variance 2 on a filtered probability space (,F,{Fn},P), where Fn=(Zk,0kn) for all n1. Define Sn=Z1+Z2++Zn, and Xn=Sn2n2 for all n1. Show that {Xn,n1} is a martingale with respect to the filtration {Fn}