Question
Use C language and recursion There is a fractal consists of infinitely many horizontal segments, which are numbered by integers started from 1. The i-th
Use C language and recursion
There is a fractal consists of infinitely many horizontal segments, which are numbered by integers started from 1. The i-th segment is represented by the range [XL[i], XR[i]], where XL[i] and XR[i] are integers, and XL[i] XR[i]. Here is the algorithm to construct the fractal. The fractal starts with the first segment XL[1] = XR[1] = 1. Then, for every iteration, it follows these steps:
Supposed that segment 1 to K have been generated by the previous iteration.
First, add (K + 1)-th segment where XL[K + 1] = 1 and XR[K + 1] = K + 1.
Then, the next K segments are the translation of the first K segments. Formally, for all segment i where 1 i K, add (K +1+i)-th segment where XL[K +1+i] = XL[i] + K and XR[K + 1 + i] = XR[i] + K.
At first, there is only segment 1. After the first iteration, there will be 1 + 1 + 1 = 3 segments. After the second iteration, there will be 3 + 1 + 3 = 7 segments. After the third iteration, there will be 7+1+7 = 15 segments. By repeating the iteration infinitely many times, the whole fractal can be created.
Since it is impossible to create a program which output infinitely many segments, it is only asked to output XL[i] and XR[i] for all segments i where A i B.
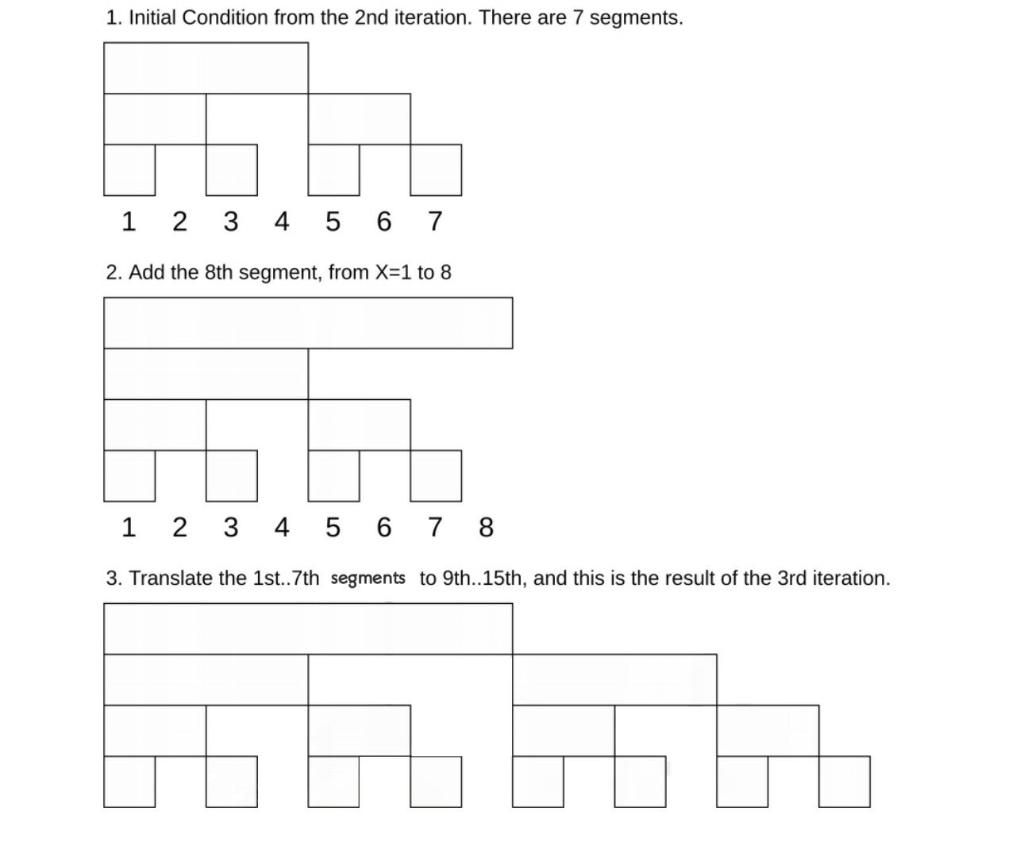
The following illustration is the step by step of creating the segments from the second iteration to the third iteration.
Format Input
A single line consists of 2 integers, A and B.
Format Output
Output (B A + 1) lines. Each line consists of 2 integers, which describes the asked
segments.
Constraints
1 A B 10^9
1 (B A + 1) 3 10^4
Sample Input 1 (standard input)
1 16
Sample Output 1 (standard output)
1 1
1 2
3 3
1 4
5 5
5 6
7 7
1 8
9 9
9 10
11 11
9 12
13 13
13 14
15 15
1 16
Sample Input 2 (standard input)
999999994 1000000000
Sample Output 2 (standard output)
999999993 999999994
999999995 999999995
999999993 999999996
999999997 999999997
999999997 999999998
999999999 999999999
999999489 1000000000
Explanation
The first illustration represents the output in the first sample.
1. Initial Condition from the 2nd iteration. There are 7 segments. 1 2 3 4 5 6 7 2. Add the 8th segment, from X=1 to 8 1 2 3 4 5 6 7 8 3. Translate the 1st.. 7th segments to 9th..15th, and this is the result of the 3rd iteration. 1. Initial Condition from the 2nd iteration. There are 7 segments. 1 2 3 4 5 6 7 2. Add the 8th segment, from X=1 to 8 1 2 3 4 5 6 7 8 3. Translate the 1st.. 7th segments to 9th..15th, and this is the result of the 3rd iterationStep by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


