Use C++

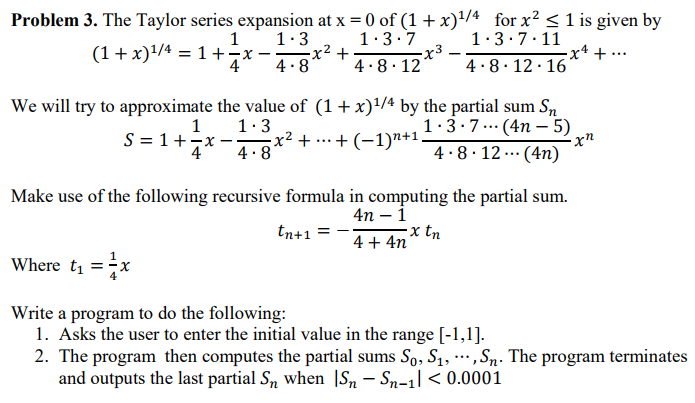
Problem 3. The Taylor series expansion at x 0 of (1x)1/4 for x2 3 1 is given by (1+": 1 + x-4-8x2 + 4-8 . 12x5-4-8-12-16 1 1.3 1.3-7 1.3-7-11 We will try to approximate the value of (1 x)1/4 by the partial sum S, 11.3 1 . 3 . 7 (4n-5) 4-8-12. (4n) -X2 + + (-1)n+1 Make use of the following recursive formula in computing the partial sum. Where t1x Write a program to do the following 1. Asks the user to enter the initial value in the range [-1,1] 2. The program then computes the partial sums So, S1, .- , Sn. The program terminates and outputs the last partial Sn when |Sn - Sn-i0.0001 Problem 3. The Taylor series expansion at x 0 of (1x)1/4 for x2 3 1 is given by (1+": 1 + x-4-8x2 + 4-8 . 12x5-4-8-12-16 1 1.3 1.3-7 1.3-7-11 We will try to approximate the value of (1 x)1/4 by the partial sum S, 11.3 1 . 3 . 7 (4n-5) 4-8-12. (4n) -X2 + + (-1)n+1 Make use of the following recursive formula in computing the partial sum. Where t1x Write a program to do the following 1. Asks the user to enter the initial value in the range [-1,1] 2. The program then computes the partial sums So, S1, .- , Sn. The program terminates and outputs the last partial Sn when |Sn - Sn-i0.0001