Use Python

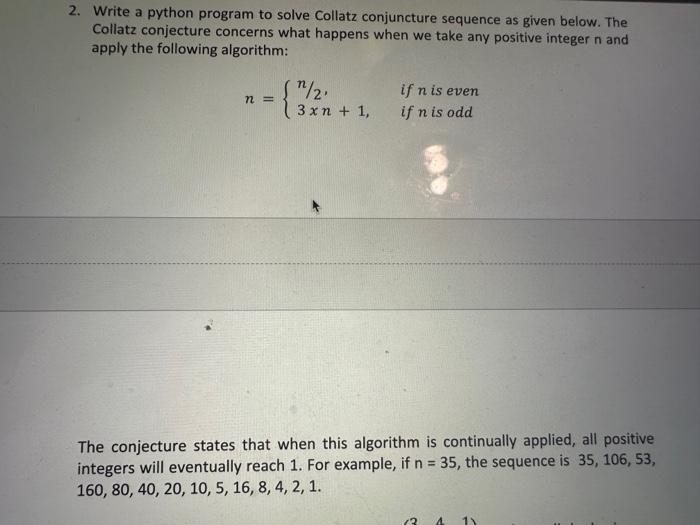
1. In this part of the assignment, you will work with file 1/0 operations, dictionaries, Numpy arrays and visualization with Matplotlib. You will write a simple Python script that will read a file named test.txt. You can hard code this into your program for convenience. A sample file is given on Canvas for your reference. Your program should read a text file and print a histogram of the letters present in the document. The code must have the histogram as both a dictionary, where the keys are letters and the values are the corresponding frequencies, as well as a N inpy array, where each index corresponds to a letter in alphabetical order. For examrle, the letter 'a' corresponds to index 0 , ' b ' to index 1 , etc. An example of a histogram for a line is given below: Input: The quick brown fox jumps over the lazy dog Output: {a:1,b:1,c:1,d:1,e:3, ' i:1,j0:1,k:1,1:1,m:1,n:1, 0:4,p:1,q:1,r: 2, 's': 1, 't': :2,u:2,vv:1,w:m:1,x:1, Y:1,:1} 2. Write a python program to solve Collatz conjuncture sequence as given below. The Collatz conjecture concerns what happens when we take any positive integer n and apply the following algorithm: n={n/2,2vn+1ifnisevenifnisodd 2. Write a python program to solve Collatz conjuncture sequence as given below. The Collatz conjecture concerns what happens when we take any positive integer n and apply the following algorithm: n={n/2,3xn+1,ifnisevenifnisodd The conjecture states that when this algorithm is continually applied, all positive integers will eventually reach 1 . For example, if n=35, the sequence is 35,106,53, 160,80,40,20,10,5,16,8,4,2,1. 3. Create array x and y, where x=(1,4,3) and y=(324719). Perform all the basics mathematical operations like addition, subtraction, multiplication, transpose, xy and division. 4. Write a function mymax(x) to return the maximum (largest) value in x without using any built-in functions. 5. Let Q(x) be the quadratic equation Q(x)=ax2+b+c=0 for some scalar values a, b, and c. A root of Q(x) is an r such that Q(r)=0. The two roots of a quadratic equation can be describsd by the quadratic formula, which is r=2abb24ac A quadratic equation has either two real roots (i.e., b2>4ac ), two imaginary roots (i.e., b2