Use R software

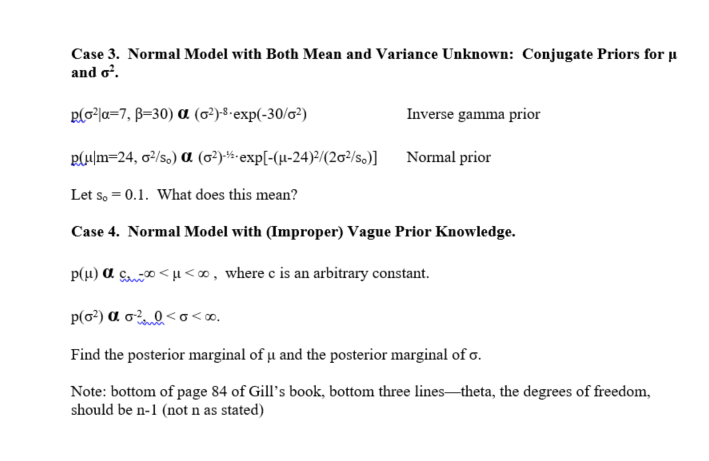
Let X1, X2, me X10 be the fuel economy (mpg) for ten randomly chosen owners of a 2017 Chevrolet Equinox with a 2.4L DOHC engine. The fuel economy is measured by dividing the total miles accumulated in a specified month by the total fuel consumed that month. This measure is an in use fuel economy in contrast to the fuel economy assessed by the EPA and noted on the window sticker for the vehicle. The ten measured values are (in mpg): 25.652 23.995 23.176 22.989 24.370 26.456 28.816 26.230 26.899 25.586 The sample mean is 25.417 mpg and the sample standard deviation is 1.814 mpg. We are going to examine four cases (assumptions) about the population of the 2017 Equinoxs, In all cases, the data are assumed to follow a normal distribution. For each case: (a) State and plot the prior probability density. (b) Calculate and plot the posterior probability density (plot together with (a)). Calculate the 0.01, 0.10, 0.25, 0.50, 0.75, 0.90, and 0.99 percentiles of the posterior distribution. (d) Calculate a 90% credible interval for the quantity of interest. (e) Write a brief conclusion comparing the prior and posterior distributions. Case 1. Normal Model with Known Variance: Conjugate Prior for the mean, u. Suppose a standard deviation = 2 and the unknown parameter of interest is the mean of this distribution, ut, which is given a conjugate normal prior with assigned parameters m= 24 and 52 = 4. Case 2. Normal Model with Mean Known: Conjugate Prior for the Variance o?. Suppose a known mean u = 25 and the unknown parameter of interest is the variance 62 which is given a conjugate inverse gamma prior with assigned parameters a = 7 and B = 30. Case 3. Normal Model with Both Mean and Variance Unknown: Conjugate Priors for u and o. p(02/a=7, B=30) (62)-8.exp(-30/02) Inverse gamma prior p(um=24, 6-/s.) a (62)-"-exp[-(u-24)/(202/s.)] Normal prior Let so = 0.1. What does this mean? Case 4. Normal Model with (Improper) Vague Prior Knowledge. p(u) a Como