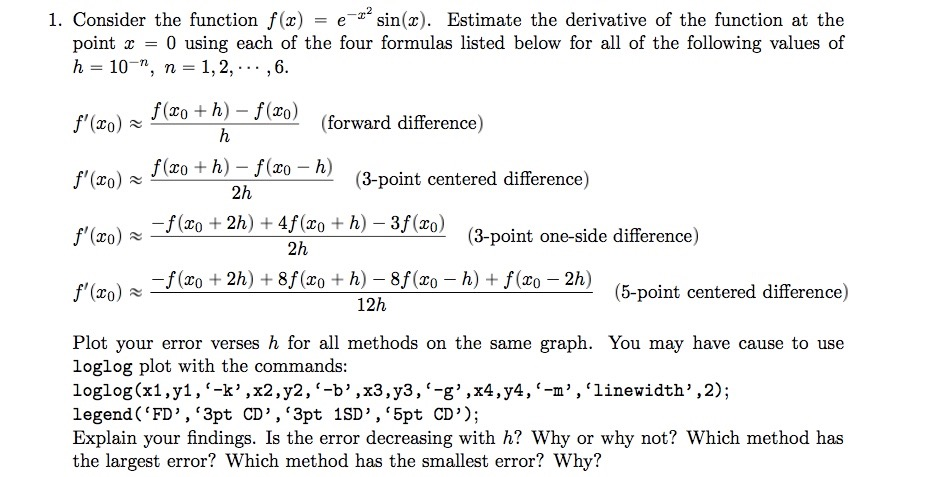
Using Mathlab!

Consider the function f(x) = e^-x^2 sin (x). Estimate the derivative of the function at the point x = 0 using each of the four formulas listed below for all of the following values of h= 10^n, n = 1, 2, ..., 6. f'(x_0 + h) - f(x_0)/h (forward difference) f'(x_0) f(x_0 + h) - f(x_0 - h)/2 h (3-point centered difference) f'(x_0) -f(x_0 + 2h) + 4f(x_0 + h) - 3f(x_0)/2h f'(x_0) -f(x_0 + 2h) + 8f(x_0 + h) - 8f(_0 - h)) + f(x_0 - 2h)/12h Plot your error verses ft for all methods on the same graph. You may have cause to use loglog plot with the commands: loglog loglog(x1, y1, '-k', x2, y2, '-b', x3, y3, '-g', x4, y4, '-m', linewidth', 2); legend ('FD', '3pt CD', '3pt 1SD', '5pt CD'); Explain your findings. Is the error decreasing with ft? Why or why not? Which method has the largest error? Which method has the smallest error? Why