Using MATLAB, Create a script that successfully answers the questions provided. Please show script and notes, will rate for correct answer.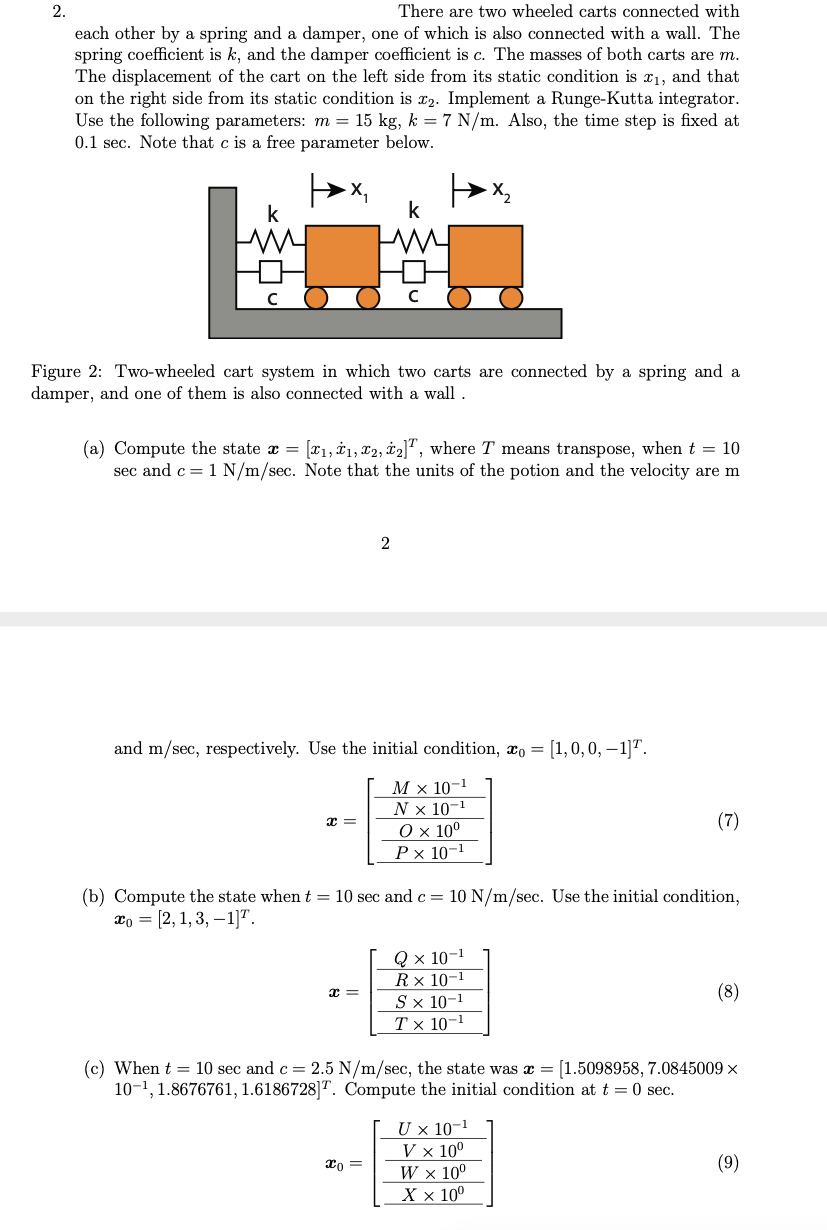

Please use Matlab

There are two wheeled carts connected with each other by a spring and a damper, one of which is also connected with a wall. The spring coefficient is k, and the damper coefficient is c. The masses of both carts are m. The displacement of the cart on the left side from its static condition is x1, and that on the right side from its static condition is 22. Implement a Runge-Kutta integrator. Use the following parameters: m = 15 kg, k = 7 N/m. Also, the time step is fixed at 0.1 sec. Note that c is a free parameter below. VL Figure 2: Two-wheeled cart system in which two carts are connected by a spring and a damper, and one of them is also connected with a wall. (a) Compute the state x = (x1, 01, 22,02)", where T means transpose, when t = 10 sec and c=1 N/m/sec. Note that the units of the potion and the velocity are m and m/sec, respectively. Use the initial condition, Xo = (1,0,0,-1)". = M X 10-1 N x 10-1 0 x 10 P x 10-1 (b) Compute the state when t = 10 sec and c = 10 N/m/sec. Use the initial condition, 20 = [2, 1, 3, -1]". Q x 10- 11 Rx 10-1 S x 10-1 T x 10-1 (8) | (c) When t = 10 sec and c= 2.5 N/m/sec, the state was x = (1.5098958, 7.0845009 X 10-1.1.8676761, 1.6186728]T. Compute the initial condition at t=0 sec. To = U x 10-1 V x 10 W x 10 X x 10 There are two wheeled carts connected with each other by a spring and a damper, one of which is also connected with a wall. The spring coefficient is k, and the damper coefficient is c. The masses of both carts are m. The displacement of the cart on the left side from its static condition is x1, and that on the right side from its static condition is 22. Implement a Runge-Kutta integrator. Use the following parameters: m = 15 kg, k = 7 N/m. Also, the time step is fixed at 0.1 sec. Note that c is a free parameter below. VL Figure 2: Two-wheeled cart system in which two carts are connected by a spring and a damper, and one of them is also connected with a wall. (a) Compute the state x = (x1, 01, 22,02)", where T means transpose, when t = 10 sec and c=1 N/m/sec. Note that the units of the potion and the velocity are m and m/sec, respectively. Use the initial condition, Xo = (1,0,0,-1)". = M X 10-1 N x 10-1 0 x 10 P x 10-1 (b) Compute the state when t = 10 sec and c = 10 N/m/sec. Use the initial condition, 20 = [2, 1, 3, -1]". Q x 10- 11 Rx 10-1 S x 10-1 T x 10-1 (8) | (c) When t = 10 sec and c= 2.5 N/m/sec, the state was x = (1.5098958, 7.0845009 X 10-1.1.8676761, 1.6186728]T. Compute the initial condition at t=0 sec. To = U x 10-1 V x 10 W x 10 X x 10