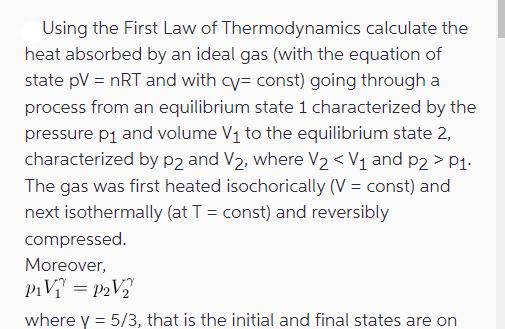
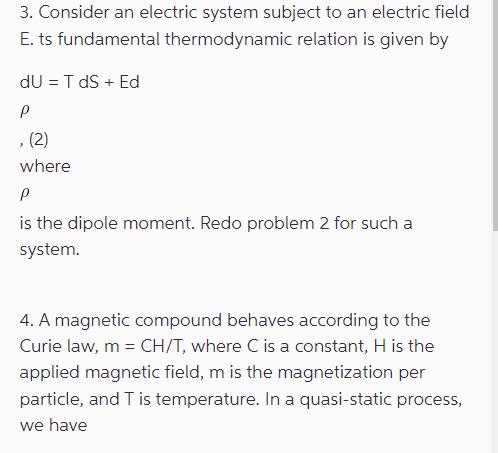
Using the First Law of Thermodynamics calculate the heat absorbed by an ideal gas (with the equation of state pV = nRT and with cy= const) going through a process from an equilibrium state 1 characterized by the pressure p and volume V to the equilibrium state 2, characterized by p2 and V, where V < V and p2 > P1. The gas was first heated isochorically (V = const) and next isothermally (at T = const) and reversibly compressed. Moreover, PV=pV where y = 5/3, that is the initial and final states are on where y = 5/3, that is the initial and final states are on the same adiabath. 2. Consider a magnetic system subject to a magnetic field H. Its fundamental thermodynamic relation is given by dU = T dS + oHdM (1) where o is the vacuum magnetic permeability and M is the total magnetic moment of such a system. Derive the respective thermodynamic potentials for such a system, as well as the heat capacities at constant magnetic moment and constant magnetic field. 3. Consider an electric system subject to an electric field E. ts fundamental thermodynamic relation is given by dU = T dS + Ed P , (2) where P is the dipole moment. Redo problem 2 for such a system. 4. A magnetic compound behaves according to the Curie law, m = CH/T, where C is a constant, H is the applied magnetic field, m is the magnetization per particle, and T is temperature. In a quasi-static process, we have du T ds + Hdm, (3) where u = u(s, m) plays the role of an internal energy. For an infinitesimal adiabatic process, show that we can write AT = CH CHT (4) where CH is the specific heat at constant magnetic field.