Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Using the P(x) = 0.90 P(y) = 0.05 P(z|x,y) = 0.25 P(z|x, y)=0.85 P(z|-x,y) = 0.85 P(z|-x, y)=0.85 What is the probability of P(-x|z)?
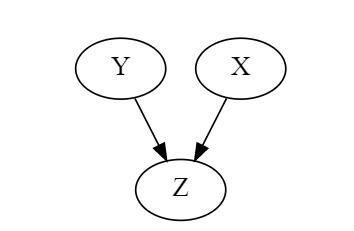
Using the P(x) = 0.90 P(y) = 0.05 P(z|x,y) = 0.25 P(z|x, y)=0.85 P(z|-x,y) = 0.85 P(z|-x, y)=0.85 What is the probability of P(-x|z)? Bayesian network with the following conditional probability Activ Go to Y Z X
Step by Step Solution
There are 3 Steps involved in it
Step: 1
The problem presented is a classic example of Bayesian inference and we want to calculate the probab...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
Understanding Basic Statistics
Authors: Charles Henry Brase, Corrinne Pellillo Brase
6th Edition
978-1133525097, 1133525091, 1111827028, 978-1133110316, 1133110312, 978-1111827021
