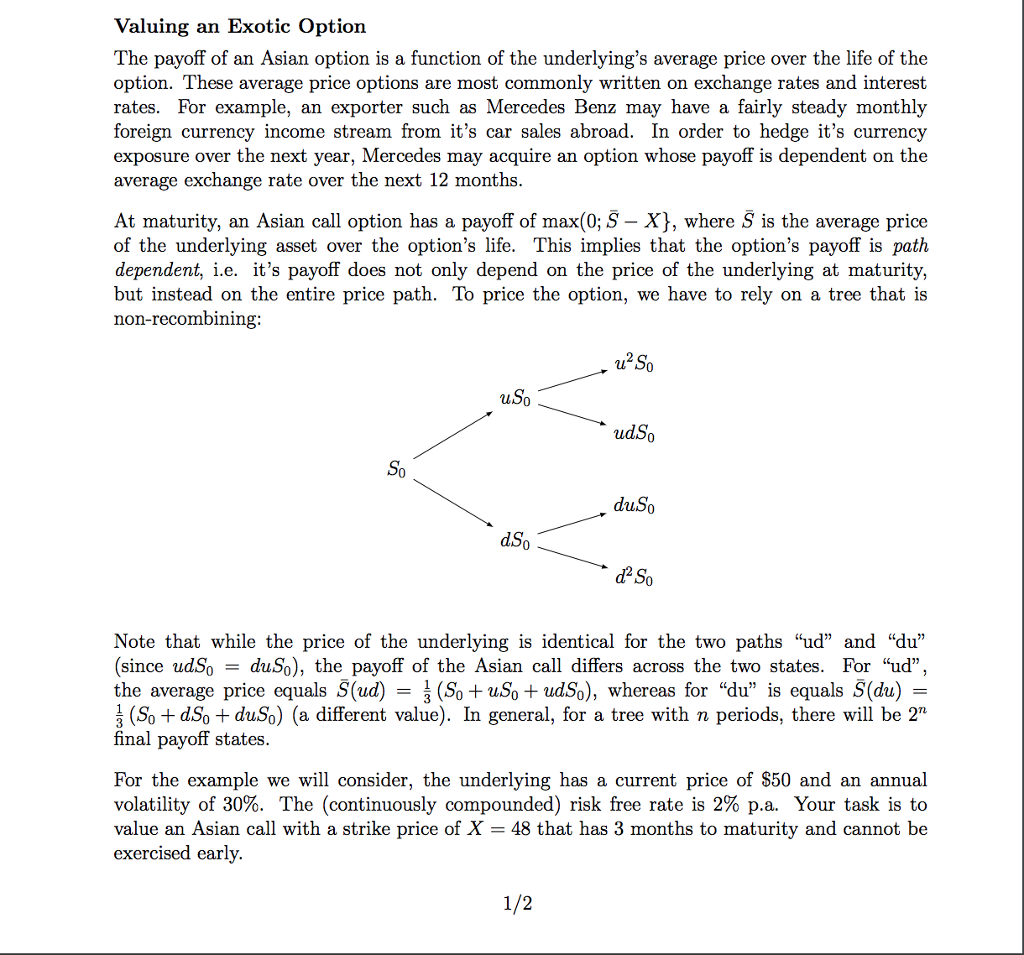

Valuing an Exotic Option The payoff of an Asian option is a function of the underlying's average price over the life of the option. These average price options are most commonly written on exchange rates and interest rates. For example, an exporter such as Mercedes Benz may have a fairly steady monthly foreign currency income stream from it's car sales abroad. In order to hedge it's currency exposure over the next year, Mercedes may acquire an option whose payoff is dependent on the average exchange rate over the next 12 months At maturity, an Asian call option has a payoff of max(0; S - X), where is the average price of the underlying asset over the option's life. This implies that the option's payoff is path dependent, i.e. it's payoff does not only depend on the price of the underlying at maturity, but instead on the entire price path. To price the option, we have to rely on a tree that is non-recombining: u2 So So 0 dS 0 d2 So Note that while the price of the underlying is identical for the two paths "ud" and "du" (since udSo = dus), the payoff of the Asian call differs across the two states. For "ud", the average price equals S(ud)SouSo udSo), whereas for "du" is equals S(du) (Ss+ dSo + duSJ (a different value). In general, for a tree with n periods, there will be 2n final payoff states For the example we will consider, the underlying has a current price of S50 and an annual volatility of 30%. The (continuously compounded) risk free rate is 2% p.a. Your task is to value an Asian call with a strike price of X -48 that has 3 months to maturity and cannot be exercised early. Valuing an Exotic Option The payoff of an Asian option is a function of the underlying's average price over the life of the option. These average price options are most commonly written on exchange rates and interest rates. For example, an exporter such as Mercedes Benz may have a fairly steady monthly foreign currency income stream from it's car sales abroad. In order to hedge it's currency exposure over the next year, Mercedes may acquire an option whose payoff is dependent on the average exchange rate over the next 12 months At maturity, an Asian call option has a payoff of max(0; S - X), where is the average price of the underlying asset over the option's life. This implies that the option's payoff is path dependent, i.e. it's payoff does not only depend on the price of the underlying at maturity, but instead on the entire price path. To price the option, we have to rely on a tree that is non-recombining: u2 So So 0 dS 0 d2 So Note that while the price of the underlying is identical for the two paths "ud" and "du" (since udSo = dus), the payoff of the Asian call differs across the two states. For "ud", the average price equals S(ud)SouSo udSo), whereas for "du" is equals S(du) (Ss+ dSo + duSJ (a different value). In general, for a tree with n periods, there will be 2n final payoff states For the example we will consider, the underlying has a current price of S50 and an annual volatility of 30%. The (continuously compounded) risk free rate is 2% p.a. Your task is to value an Asian call with a strike price of X -48 that has 3 months to maturity and cannot be exercised early








