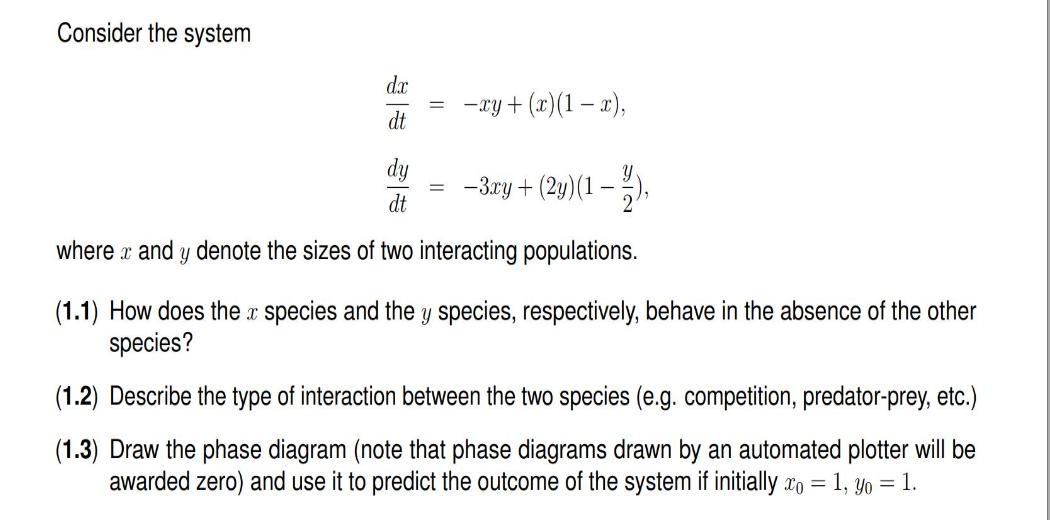
Consider the system dx dt - xy + (x)(1-x), dy dt where and y denote the sizes of two interacting populations. = -3xy + (2y) (1 Y (1.1) How does the species and the y species, respectively, behave in the absence of the other species? (1.2) Describe the type of interaction between the two species (e.g. competition, predator-prey, etc.) (1.3) Draw the phase diagram (note that phase diagrams drawn by an automated plotter will be awarded zero) and use it to predict the outcome of the system if initially xo = 1, yo = 1.