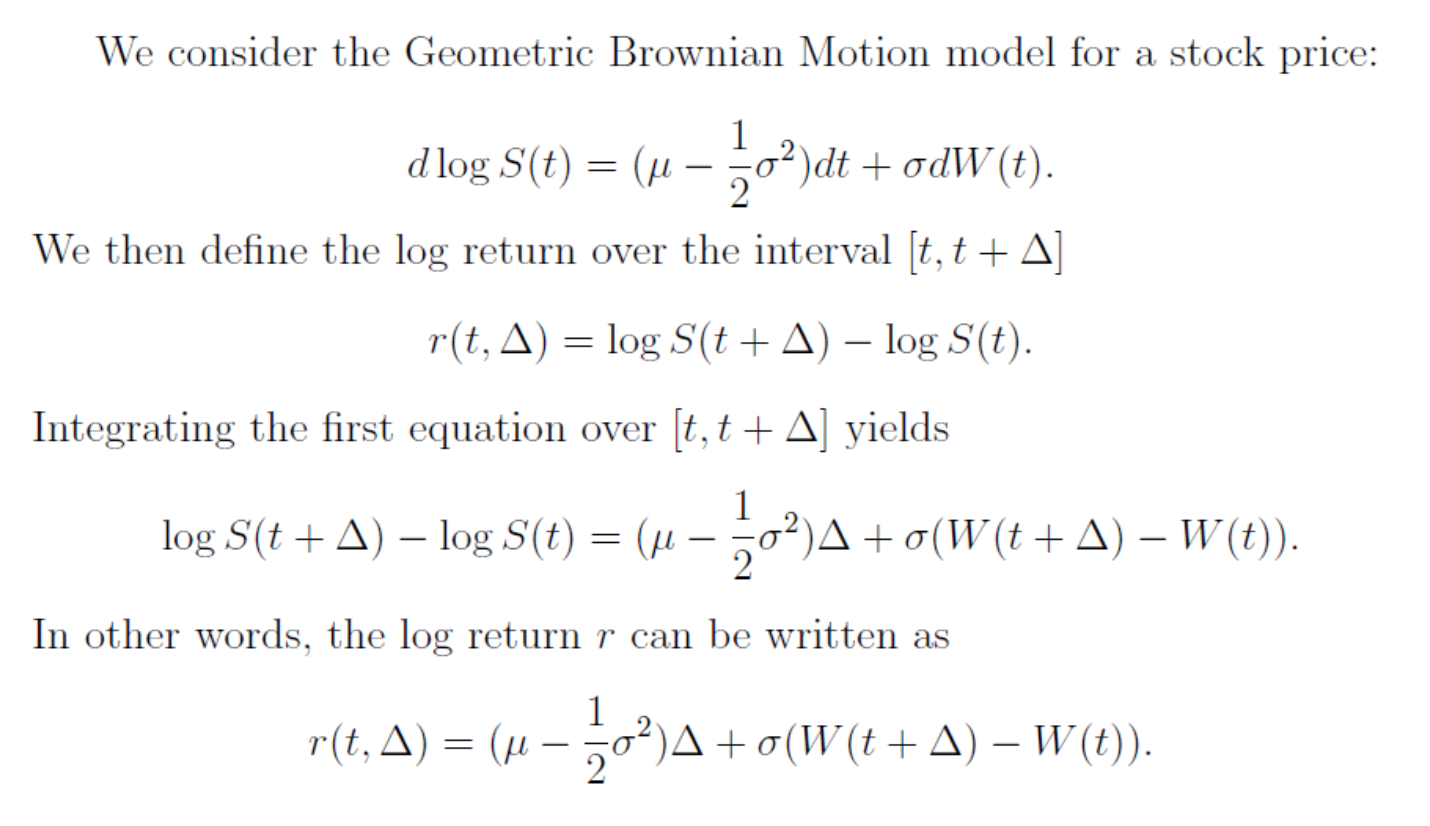
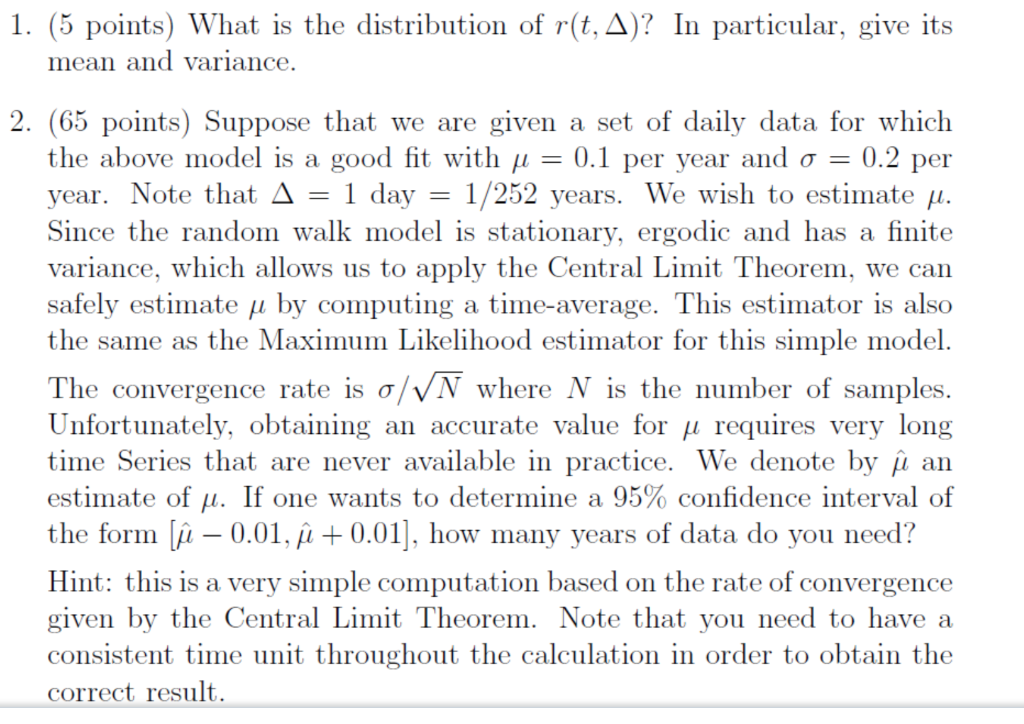
We consider the Geometric Brownian Motion model for a stock price: dlogS(t)=(212)dt+dW(t). We then define the log return over the interval [t,t+] r(t,)=logS(t+)logS(t). Integrating the first equation over [t,t+] yields logS(t+)logS(t)=(212)+(W(t+)W(t)). In other words, the log return r can be written as r(t,)=(212)+(W(t+)W(t)). 1. (5 points) What is the distribution of r(t,) ? In particular, give its mean and variance. 2. (65 points) Suppose that we are given a set of daily data for which the above model is a good fit with =0.1 per year and =0.2 per year. Note that =1 day =1/252 years. We wish to estimate . Since the random walk model is stationary, ergodic and has a finite variance, which allows us to apply the Central Limit Theorem, we can safely estimate by computing a time-average. This estimator is also the same as the Maximum Likelihood estimator for this simple model. The convergence rate is /N where N is the number of samples. Unfortunately, obtaining an accurate value for requires very long time Series that are never available in practice. We denote by ^ an estimate of . If one wants to determine a 95% confidence interval of the form [^0.01,^+0.01], how many years of data do you need? Hint: this is a very simple computation based on the rate of convergence given by the Central Limit Theorem. Note that you need to have a consistent time unit throughout the calculation in order to obtain the correct result. We consider the Geometric Brownian Motion model for a stock price: dlogS(t)=(212)dt+dW(t). We then define the log return over the interval [t,t+] r(t,)=logS(t+)logS(t). Integrating the first equation over [t,t+] yields logS(t+)logS(t)=(212)+(W(t+)W(t)). In other words, the log return r can be written as r(t,)=(212)+(W(t+)W(t)). 1. (5 points) What is the distribution of r(t,) ? In particular, give its mean and variance. 2. (65 points) Suppose that we are given a set of daily data for which the above model is a good fit with =0.1 per year and =0.2 per year. Note that =1 day =1/252 years. We wish to estimate . Since the random walk model is stationary, ergodic and has a finite variance, which allows us to apply the Central Limit Theorem, we can safely estimate by computing a time-average. This estimator is also the same as the Maximum Likelihood estimator for this simple model. The convergence rate is /N where N is the number of samples. Unfortunately, obtaining an accurate value for requires very long time Series that are never available in practice. We denote by ^ an estimate of . If one wants to determine a 95% confidence interval of the form [^0.01,^+0.01], how many years of data do you need? Hint: this is a very simple computation based on the rate of convergence given by the Central Limit Theorem. Note that you need to have a consistent time unit throughout the calculation in order to obtain the correct result








