Answered step by step
Verified Expert Solution
Question
1 Approved Answer
We try to build a deeper understanding of the endogeneity issue in the regression model y = a + bx + . Suppose that

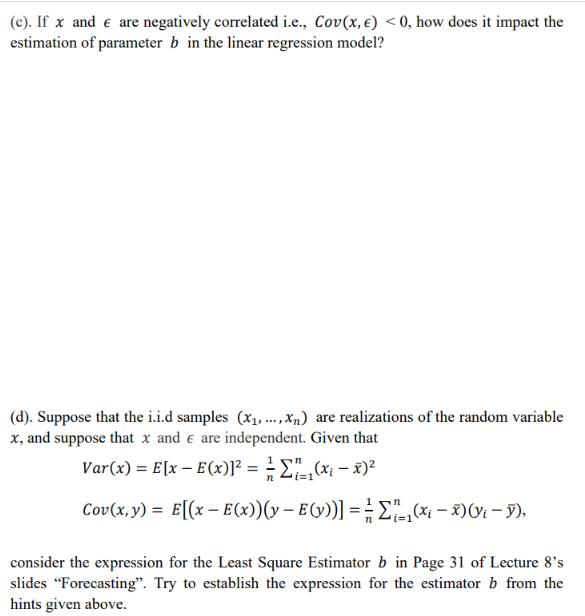
We try to build a deeper understanding of the endogeneity issue in the regression model y = a + bx + . Suppose that x and are random variables, and parameters a and b are constants. Cov(x,y)-Cov(x,E) (a). Show that b Var (x) = Hint: the covariance of the random variables Cov(x, y) has the following properties Cov(x, y) = Cov(x, a + bx + ) = Cov(x, a) + Cov(x, bx) + Cov(x, e), Cov(x, bx)=bVar(x). (b). If x and e are independent, what is Cov(x, e)? (c). If x and are negatively correlated i.e., Cov(x, e) < 0, how does it impact the estimation of parameter b in the linear regression model? (d). Suppose that the i.i.d samples (x,...,xn) are realizations of the random variable x, and suppose that x and e are independent. Given that Var(x) = E[x - E(x)] = (x - x) i=1 Cov(x, y) = E[(x - E(x))(y-E(y))] = (x - x)(y - y), consider the expression for the Least Square Estimator b in Page 31 of Lecture 8's slides "Forecasting". Try to establish the expression for the estimator b from the hints given above.
Step by Step Solution
★★★★★
3.52 Rating (155 Votes )
There are 3 Steps involved in it
Step: 1
a To show that b Covxy Covxe Varx well use the properties of covariance mentioned in the hint We sta...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


