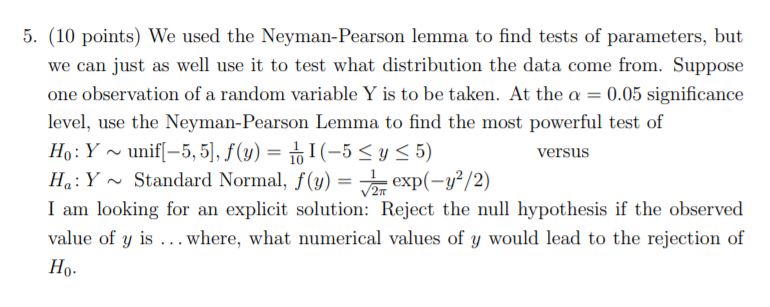
We used the Neyman-Pearson lemma to find tests of parameters, but we can just as well use it to test what distribution the data come from. Suppose one observation of a random variable Y is to be taken. At the ? = 0.05 significance level, use the Neyman-Pearson Lemma to find the most powerful test of H0 : Y ? unif[?5, 5], f(y) = 1 10 I (?5 ? y ? 5) versus Ha : Y ? Standard Normal, f(y) = ? 1 2? exp(?y 2/2) I am looking for an explicit solution: Reject the null hypothesis if the observed value of y is . . . where, what numerical values of y would lead to the rejection of H0.

\f