What did I get wrong on these?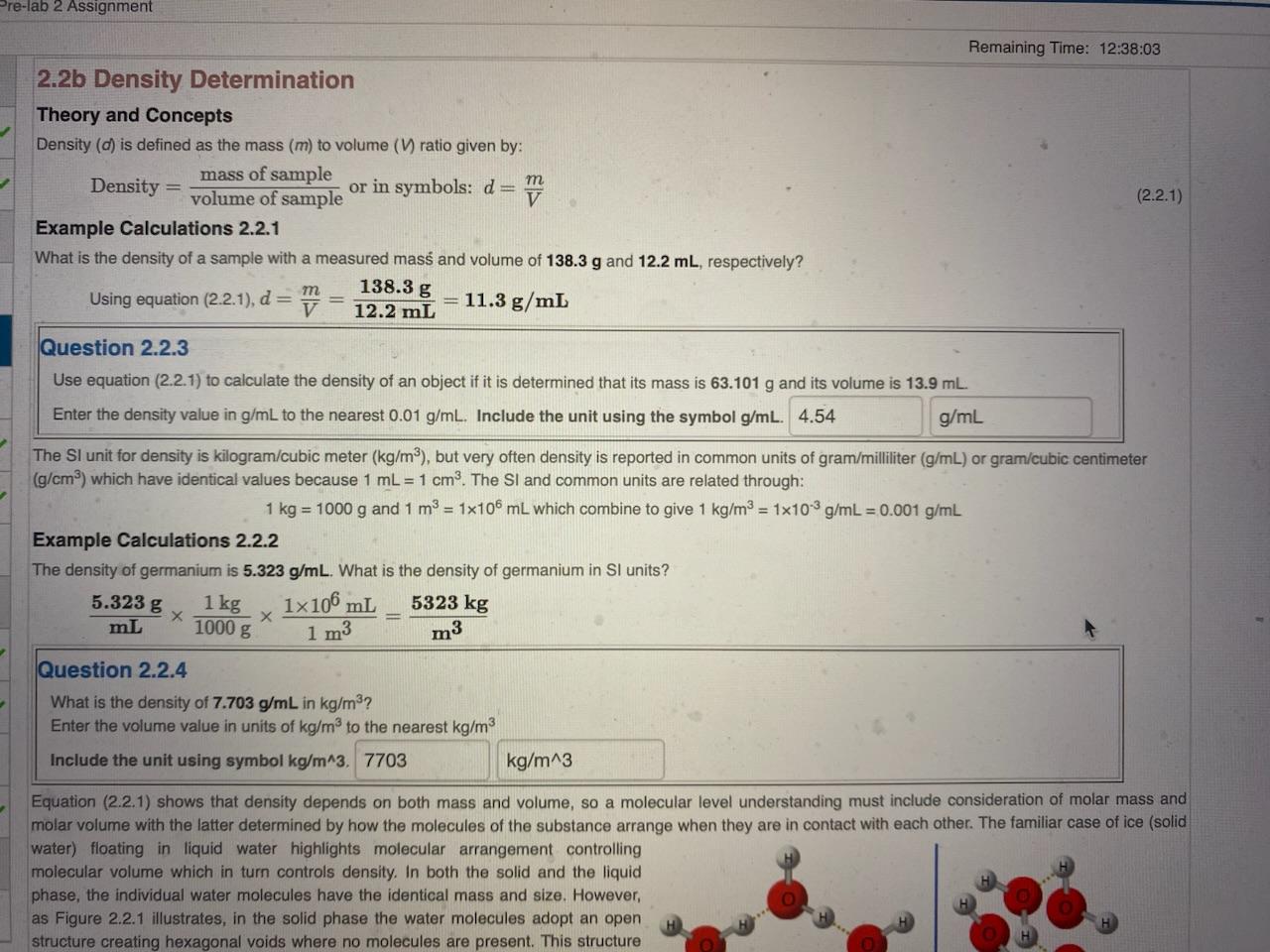
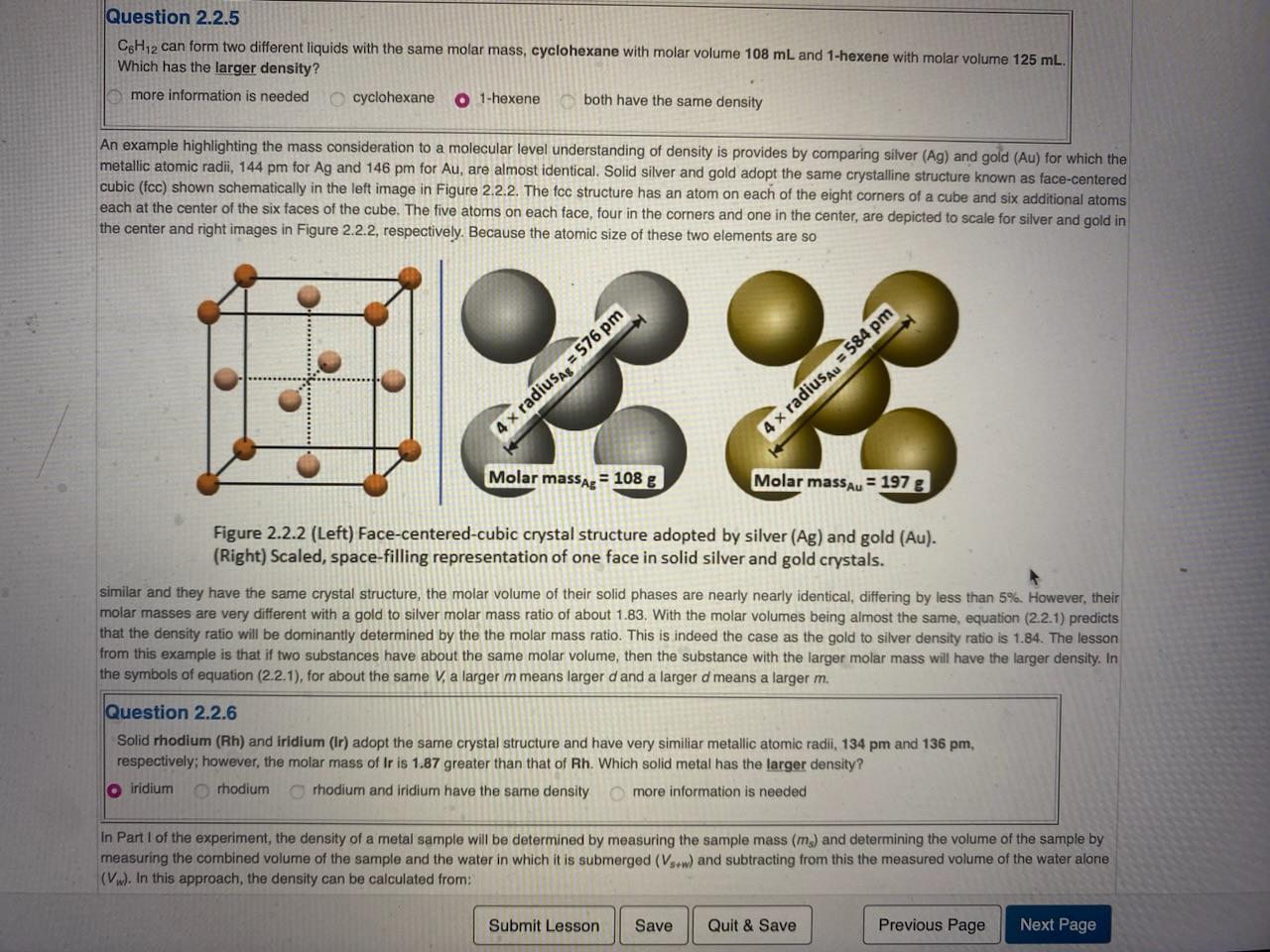
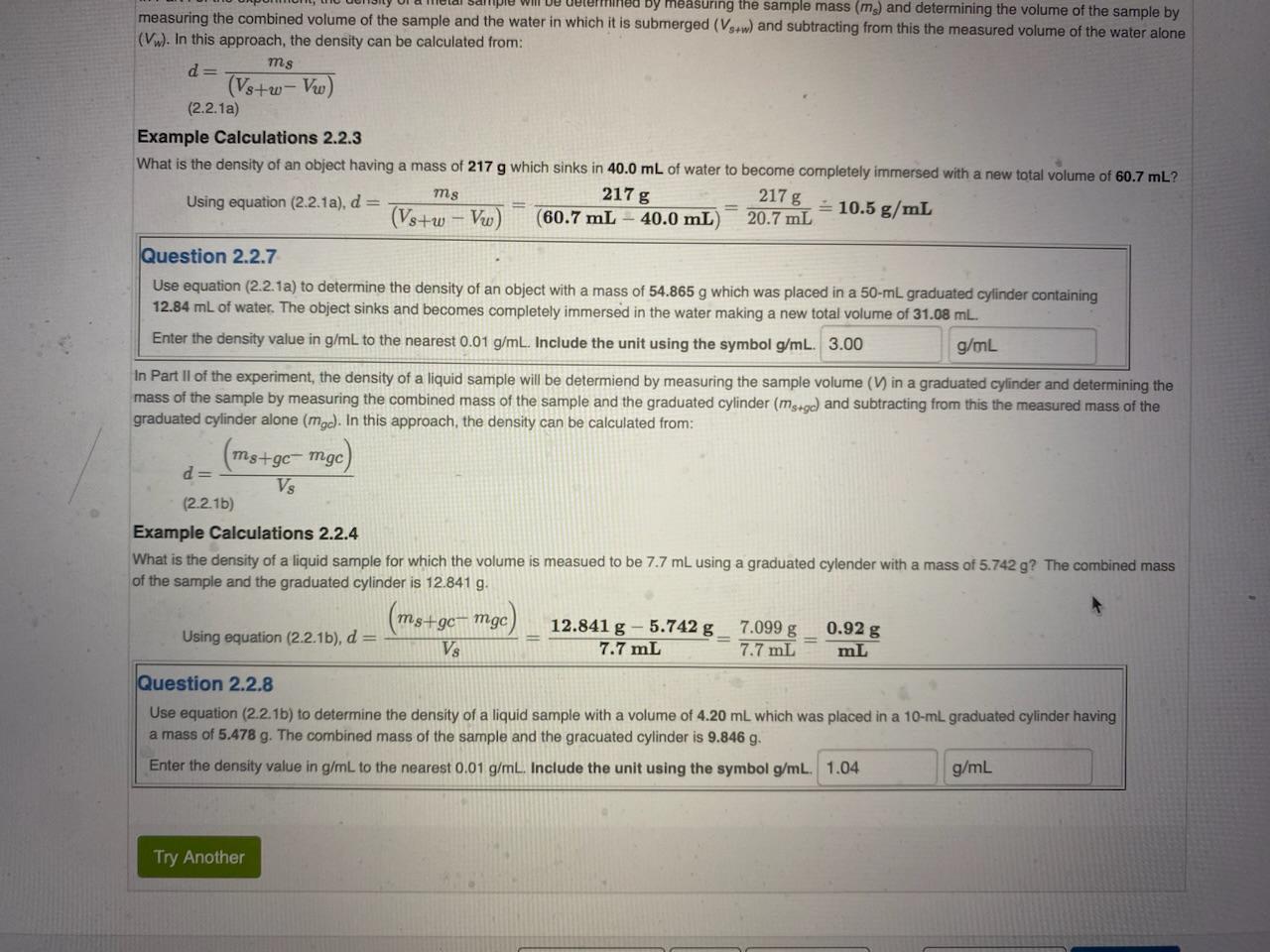
Remaining Time: 12:38:03 2.2b Density Determination Theory and Concepts Density (d) is defined as the mass (m) to volume (V) ratio given by: Density =volumeofsamplemassofsample or in symbols: d=Vm (2.2.1) Example Calculations 2.2.1 What is the density of a sample with a measured mass and volume of 138.3g and 12.2mL, respectively? Using equation (2.2.1), d=Vm=12.2mL138.3g=11.3g/mL Question 2.2.3 Use equation (2.2.1) to calculate the density of an object if it is determined that its mass is 63.101g and its volume is 13.9mL Enter the density value in g/mL to the nearest 0.01g/mL. Include the unit using the symbol g/mL. The SI unit for density is kilogram/cubic meter (kg/m3), but very often density is reported in common units of gram/milliliter (g/mL) or gram/cubic centimeter (g/cm3 ) which have identical values because 1mL=1cm3. The SI and common units are related through: 1kg=1000g and 1m3=1106mL which combine to give 1kg/m3=1103g/mL=0.001g/mL Example Calculations 2.2.2 The density of germanium is 5.323g/mL. What is the density of germanium in SI units? mL5.323g1000g1kg1m31106mL=m35323kg Question 2.2.4 What is the density of 7.703g/mL in kg/m3 ? Enter the volume value in units of kg/m3 to the nearest kg/m3 Include the unit using symbol kg/m3. Equation (2.2.1) shows that density depends on both mass and volume, so a molecular level understanding must include consideration of molar mass and molar volume with the latter determined by how the molecules of the substance arrange when they are in contact with each other. The familiar case of ice (solid water) floating in liquid water highlights molecular arrangement controlling molecular volume which in turn controls density. In both the solid and the liquid phase, the individual water molecules have the identical mass and size. However, as Figure 2.2.1 illustrates, in the solid phase the water molecules adopt an open structure creating hexagonal voids where no molecules are present. This structure C6H12 can form two different liquids with the same molar mass, cyclohexane with molar volume 108mL and 1 -hexene with molar volume 125mL. Which has the larger density? more information is needed cyclohexane 1-hexene both have the same density An example highlighting the mass consideration to a molecular level understanding of density is provides by comparing silver (Ag) and gold (Au) for which the metallic atomic radii, 144pm for Ag and 146pm for Au, are almost identical. Solid silver and gold adopt the same crystalline structure known as face-centered cubic (fcc) shown schematically in the left image in Figure 2.2.2. The fcc structure has an atom on each of the eight corners of a cube and six aditional atoms each at the center of the six faces of the cube. The five atoms on each face, four in the corners and one in the center, are depicted to scale for silver and gold in the center and right images in Figure 2.2.2, respectively. Because the atomic size of these two elements are so Figure 2.2.2 (Left) Face-centered-cubic crystal structure adopted by silver (Ag) and gold (Au). (Right) Scaled, space-filling representation of one face in solid silver and gold crystals. similar and they have the same crystal structure, the molar volume of their solid phases are nearly nearly identical, differing by less than 5%. However, their molar masses are very different with a gold to silver molar mass ratio of about 1.83. With the molar volumes being almost the same, equation (2.2.1) predicts that the density ratio will be dominantly determined by the the molar mass ratio. This is indeed the case as the gold to silver density ratio is 1.84. The lesson from this example is that if two substances have about the same molar volume, then the substance with the larger molar mass will have the larger density. In the symbols of equation (2.2.1), for about the same V, a larger m means larger d and a larger d means a larger m. Question 2.2.6 Solid rhodium (Rh) and iridium (Ir) adopt the same crystal structure and have very similiar metallic atomic radii, 134 pm and 136 pm, respectively; however, the molar mass of Ir is 1.87 greater than that of Rh. Which solid metal has the larger density? iridium rhodium thodium and iridium have the same density more information is needed In Part I of the experiment, the density of a metal sample will be determined by measuring the sample mass ( ms) and determining the volume of the sample by measuring the combined volume of the sample and the water in which it is submerged (Vs+w) and subtracting from this the measured volume of the water alone (Vw). In this approach, the density can be calculated from: measuring the combined volume of the sample and the water in which it is submerged (Vs+w) and subtracting frome determining the volume of the sample by (Vw). In this approach, the density can be calculated from: d=(Vs+wVw)mS(2.2.1a) Example Calculations 2.2.3 What is the density of an object having a mass of 217g which sinks in 40.0mL of water to become completely immersed with a new total volume of 60.7mL ? Usingequation(2.2.1a),d=(Vs+wVw)ms=(60.7mL40.0mL)217g=20.7mL217g=10.5g/mL Question 2.2.7 Use equation (2.2.1a) to determine the density of an object with a mass of 54.865g which was placed in a 50mL graduated cylinder containing 12.84mL of water. The object sinks and becomes completely immersed in the water making a new total volume of 31.08mL. Enter the density value in g/mL to the nearest 0.01g/mL. Include the unit using the symbol g/mL. In Part Il of the experiment, the density of a liquid sample will be determiend by measuring the sample volume (V in a graduated cylinder and determining the mass of the sample by measuring the combined mass of the sample and the graduated cylinder (ms+g) and subtracting from this the measured mass of the graduated cylinder alone (mgc). In this approach, the density can be calculated from: d=Vs(ms+gcmgc) Example Calculations 2.2.4 What is the density of a liquid sample for which the volume is measued to be 7.7mL using a graduated cylender with a mass of 5.742g ? The combined mass of the sample and the graduated cylinder is 12.841g. Usingequation(2.2.1b),d=Vs(ms+gcmgc)=7.7mL12.841g5.742g=7.7mL7.099g=mL0.92g Question 2.2.8 Use equation (2.2.1b) to determine the density of a liquid sample with a volume of 4.20mL which was placed in a 10-mL graduated cylinder having a mass of 5.478g. The combined mass of the sample and the gracuated cylinder is 9.846g. Enter the density value in g/mL to the nearest 0.01g/mL. Include the unit using the symbol g/mL









