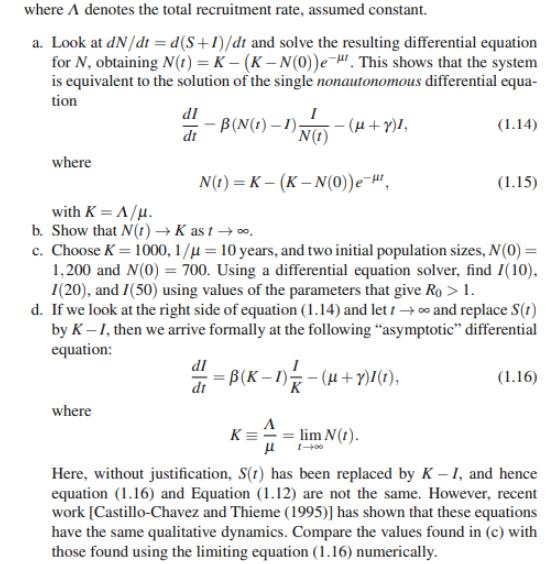
where A denotes the total recruitment rate, assumed constant. a. Look at dN/dt =d(S+1)/dt and solve the resulting differential equation for N, obtaining N(t) = K-(K-N(0))e . This shows that the system is equivalent to the solution of the single nonautonomous differential equa- tion where dl dt -B(N(1)-1); - (+ y), N(1) N(1) K-(K-N(0)) e-t, with K = A/. b. Show that N(1) Kast . where c. Choose K = 1000, 1/ = 10 years, and two initial population sizes, N(0) = 1,200 and N(0) = 700. Using a differential equation solver, find I(10), 1(20), and I(50) using values of the parameters that give Ro > 1. d. If we look at the right side of equation (1.14) and let 1 and replace S(1) by K - I, then we arrive formally at the following "asymptotic" differential equation: dl dt (1.14) = B(K 1) / 2 ( + y)1(1), (1.15) (1.16) lim N(t). K= H Here, without justification, S(1) has been replaced by K-I, and hence equation (1.16) and Equation (1.12) are not the same. However, recent work [Castillo-Chavez and Thieme (1995)] has shown that these equations have the same qualitative dynamics. Compare the values found in (c) with those found using the limiting equation (1.16) numerically.