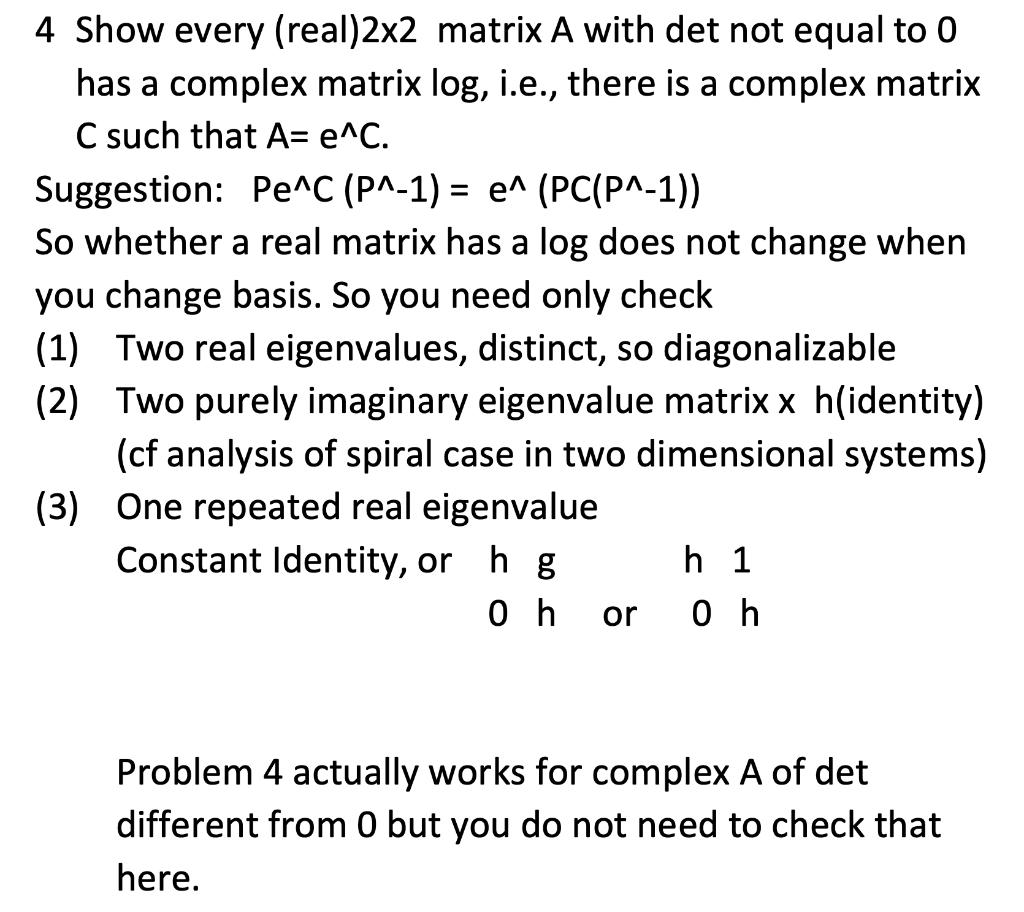
4 Show every (real)2x2 matrix A with det not equal to 0 has a complex matrix log, i.e., there is a complex matrix C such that A= e^C. Suggestion: Pe^C (P^-1) = e^ (PC(P^-1)) So whether a real matrix has a log does not change when you change basis. So you need only check (1) Two real eigenvalues, distinct, so diagonalizable (2) Two purely imaginary eigenvalue matrix x h(identity) (cf analysis of spiral case in two dimensional systems) One repeated real eigenvalue Constant Identity, or h g (3) 0 h or h 1 0 h Problem 4 actually works for complex A of det different from 0 but you do not need to check that here.