Question: where Consider the following feedback system shown below, R(s)- G(s) G(s) H(s) Gc(s) = kp S Y(s) Gp(s) = (s + 3) + 16

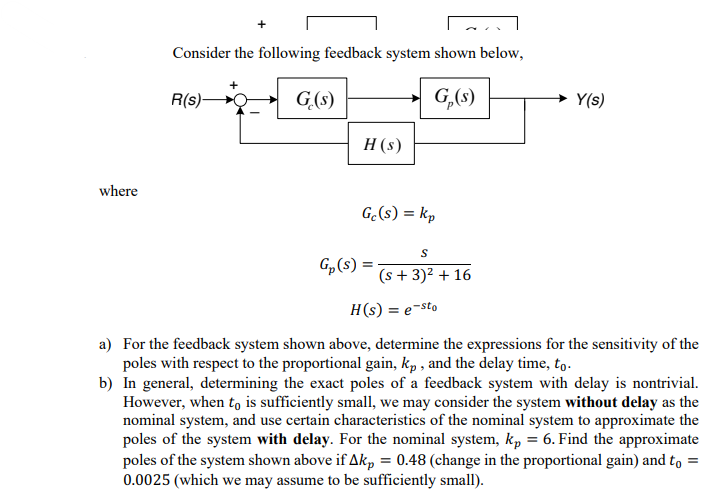
where Consider the following feedback system shown below, R(s)- G(s) G(s) H(s) Gc(s) = kp S Y(s) Gp(s) = (s + 3) + 16 H(s) = e-sto a) For the feedback system shown above, determine the expressions for the sensitivity of the poles with respect to the proportional gain, kp, and the delay time, to. b) In general, determining the exact poles of a feedback system with delay is nontrivial. However, when to is sufficiently small, we may consider the system without delay as the nominal system, and use certain characteristics of the nominal system to approximate the poles of the system with delay. For the nominal system, kp = 6. Find the approximate poles of the system shown above if Akp = 0.48 (change in the proportional gain) and to = 0.0025 (which we may assume to be sufficiently small).

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mechanical Engineering Questions!

Calculate the ending balances to update the t-accounts for the next accounting cycle. Be sure to enter all zero balances on the normal balance side and enter balances for permanent accounts! Bright,...

Q1. You have identified a market opportunity for home media players that would cater for older members of the population. Many older people have difficulty in understanding the operating principles...

CANMNMM January of this year. (a) Each item will be held in a record. Describe all the data structures that must refer to these records to implement the required functionality. Describe all the...

Q1. Consider the liquid-level control system shown in Figure 1, where R1 and R2 are water resistances, C1 and C2 are the capacitance of tanks 1 and 2, respectively. A proportional controller is added...

Give Correct ANSWERS Human-Computer Interaction (a) If you had been one of the original inventors of the WIMP interface, and engineers on the technical team had been sceptical about the advantages...

Module 6 Statistical Tests Solutions Geremy Gully PSY-845/Dr. Bielek Researchers wanted to explore self-esteem in adolescent boys and adolescent girls. Each respondent completed a 10-item self-esteem...

34 Academy of Management Perspectives A R T I C November L E S The Management of Organizational Justice by Russell Cropanzano, David E. Bowen, and Stephen W. Gilliland Executive Overview...

Gr 2 6.' ASU All GC | Co Da X G Th Yo = Ur Cc Pe DE C GC M M: | M FV a Ar Lo st G a Ar + G asuprepaz.instructure.com/courses/65975/assignments/2537446?module_item_id=5053131 0 1 Finish update :...

Algorithms in Artificial Intelligence (or, the old name: Introduction to Algorithmic Decision Making) Part 1 Based on slides by David Sarne and Lirong Xia Course Tentative Schedule Introduction...

7) [4+5+5] Consider the following level controller diagram where a feedback controller is implemented. Q h(c) (a) The following block diagram and Eqn. 1 represent the dynamics of the first order...

Imagine that your organization, a publishing company, has decided to hire an external vendor to provide diversity training. You want to begin by having the vendor conduct the training for just a...

Let X and Y correspond to the horizontal and vertical coordinates in the triangle with corners at (2,0), (0,2), and the origin. Let fX,Y (x,y) = 15/28(xy2 + y) for (x, y) inside the triangle, and...

The U - form structure used to implement a product - dflerentiation strategy has a small corporate staff. often usies temporary cross - divisional and cross - functional teams to manage the...

Two random variables X and Y have a joint probability density function given by fXY(x, y) = Kxy, 0

A random variable X has an expected value E[X], and a standard deviation oX. Another random variable Y has an expected value E[Y], and a standard deviation oY. X and Y are statistically independent....

Let the pattern P=[AABAAABA] then the LSP Array is Select one: a. 0,1,0,1,2,3,4,5 b. 0,1,0,1,2,2,3,4 c. 0,1,0,1,2,2,3,3 d. 0,1,1,2,3,3,3,4

A random variable X has an expected value E[X], and a standard deviation oX. Another random variable Y has an expected value E[Y), and a standard deviation oY. X and Y are statistically independent....

Consider the random process defines as: X(t)=B cos (2wt+3p), where B and w are constants and o is random variable X(t) can be categorized as: Select one: O a. deterministic O b. none of these O c....

In a high - context culture, what type of communication is preferred?

A series RC circuit is connected to a voltage source v i (t), and the output is the voltage across the capacitor, v o (t). (a) Find the transfer function H(s) = V o (s)/V i (s) of this filter when...

Let the transfer function of a system be Show that by defining the state-variables as v 1 (t) = y(t),v 2 (t)=Ë y(t) + a 1 y(t) b 1 x(t) we obtain a minimal state variable and output realization...

We are given a noisy signal x(t) = s(t) + Î·(t) where s(t) is the desired signal and Î·(t) is additive noise. From experience, we know that the average power of the desired...

James Kirk is a financial executive with McDowell Enterprises. Although James Kirk has not had any formal training in finance or accounting, he has a "good sense" for numbers and has helped the...

Assume that Koh ShopMart has decided to surface and maintain for 10 years a vacant lot next to one of its stores to serve as a parking lot for customers. Management is considering the following bids...

Lansbury Inc. had the statement of financial position shown below at December 31, 2018. During 2019, the following occurred. 1. Lansbury Inc. sold part of its investment portfolio for $15,000. This...