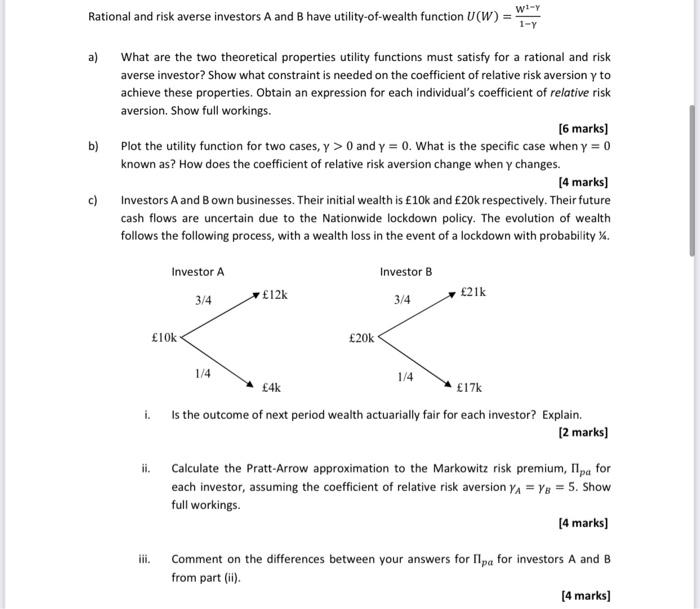
Wi-Y Rational and risk averse investors A and B have utility-of-wealth function U(W) 1-Y a) What are the two theoretical properties utility functions must satisfy for a rational and risk averse investor? Show what constraint is needed on the coefficient of relative risk aversion y to achieve these properties. Obtain an expression for each individual's coefficient of relative risk aversion. Show full workings. [6 marks) b) Plot the utility function for two cases, y > 0 and y = 0. What is the specific case when y = 0 known as? How does the coefficient of relative risk aversion change when y changes. [4 marks) c) Investors A and B own businesses. Their initial wealth is 10k and 20k respectively. Their future cash flows are uncertain due to the Nationwide lockdown policy. The evolution of wealth follows the following process, with a wealth loss in the event of a lockdown with probability %. Investor A Investor B 12k 21k 3/4 3/4 IOK 20k 1/4 1/4 4k 17k i. Is the outcome of next period wealth actuarially fair for each investor? Explain. [2 marks) ii. Calculate the Pratt-Arrow approximation to the Markowitz risk premium, II pa for each investor, assuming the coefficient of relative risk aversion YA = Y = 5. Show full workings. [4 marks) iii. Comment on the differences between your answers for Ipa for investors A and B from part (11). [4 marks) An economy contains two risky assets, A and B with the following variance-covariance matrix. " o OAB 10.2 0.11 10.1 0.1 The number of shares issued of stock A and B respectively is 100 and 200 shares, with respective prices of 10 and 20 GBP respectively. The expected return on the market is 4%, and the risk-free rate is 2%. a) Using the above information, determine the following: i What is the weight of each stock A and B in the market, based on the market capitalization of each stock. [2 marks) ii. What is the variance of the market? [2 marks] iii. What is the covariance of each stock with the market, OAM and OBM? [4 marks) iv. What is the beta of each stock? V. [2 marks] What is the expected returns on each of stocks A and B? [2 marks) vi. Plot the expected return against the beta of each stock. In addition, label the point where the B = 1, and label the corresponding expected return. What is the straight line connecting each point called? What is the slope of the line? Explain which assets or portfolios of assets should lie along this line. [3 marks) b) Given the assumptions of the Capital Asset Pricing Model, the optimal combination of the risk-free asset and risky assets held by 2 investors with different levels of risk aversion have the same Sharpe ratio. Is this statement true or false? Explain with reference to figures and equations where appropriate. (5 marks) Wi-Y Rational and risk averse investors A and B have utility-of-wealth function U(W) 1-Y a) What are the two theoretical properties utility functions must satisfy for a rational and risk averse investor? Show what constraint is needed on the coefficient of relative risk aversion y to achieve these properties. Obtain an expression for each individual's coefficient of relative risk aversion. Show full workings. [6 marks) b) Plot the utility function for two cases, y > 0 and y = 0. What is the specific case when y = 0 known as? How does the coefficient of relative risk aversion change when y changes. [4 marks) c) Investors A and B own businesses. Their initial wealth is 10k and 20k respectively. Their future cash flows are uncertain due to the Nationwide lockdown policy. The evolution of wealth follows the following process, with a wealth loss in the event of a lockdown with probability %. Investor A Investor B 12k 21k 3/4 3/4 IOK 20k 1/4 1/4 4k 17k i. Is the outcome of next period wealth actuarially fair for each investor? Explain. [2 marks) ii. Calculate the Pratt-Arrow approximation to the Markowitz risk premium, II pa for each investor, assuming the coefficient of relative risk aversion YA = Y = 5. Show full workings. [4 marks) iii. Comment on the differences between your answers for Ipa for investors A and B from part (11). [4 marks) An economy contains two risky assets, A and B with the following variance-covariance matrix. " o OAB 10.2 0.11 10.1 0.1 The number of shares issued of stock A and B respectively is 100 and 200 shares, with respective prices of 10 and 20 GBP respectively. The expected return on the market is 4%, and the risk-free rate is 2%. a) Using the above information, determine the following: i What is the weight of each stock A and B in the market, based on the market capitalization of each stock. [2 marks) ii. What is the variance of the market? [2 marks] iii. What is the covariance of each stock with the market, OAM and OBM? [4 marks) iv. What is the beta of each stock? V. [2 marks] What is the expected returns on each of stocks A and B? [2 marks) vi. Plot the expected return against the beta of each stock. In addition, label the point where the B = 1, and label the corresponding expected return. What is the straight line connecting each point called? What is the slope of the line? Explain which assets or portfolios of assets should lie along this line. [3 marks) b) Given the assumptions of the Capital Asset Pricing Model, the optimal combination of the risk-free asset and risky assets held by 2 investors with different levels of risk aversion have the same Sharpe ratio. Is this statement true or false? Explain with reference to figures and equations where appropriate