Write a MATLAB script using bisection method

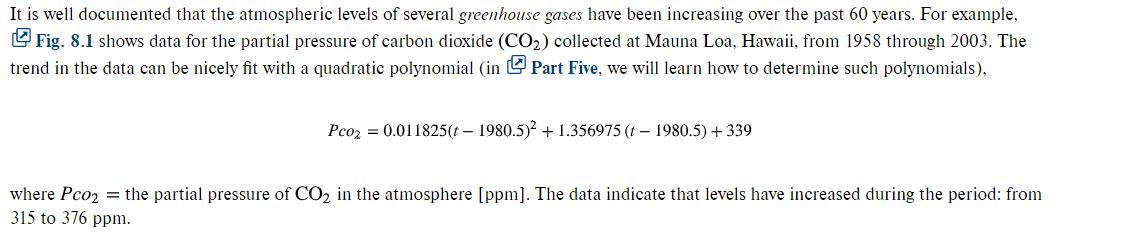
Aside from global warming, greenhouse gases can also influence atmospheric chemistry. One question that we can address is how Page 210 the carbon dioxide trend is affecting the pH of rainwater. Outside of urban and industrial areas, it is well documented that carbon dioxide is the primary determinant of the pH of the rain. pH is the measure of the activity of hydrogen ions and, therefore, of acidity. For dilute aqueous solutions, it can be computed as (8.5) pH = -log10 [H] where [H+) is the molar concentration of hydrogen ions. The following five nonlinear system of equations govern the chemistry of rainwater, (8.6) [H+] HCO3] K1 = 10 KPco2 (8.7) [H+] [CO] K = (HCO3] (8.8) Kw = [HT] [OH-] (8.9) KPco2 = 106 + [HCO3] + [203] (8.10) 0 = [HCO3] +2 [co;-] + [OH-] - [h*] where Ku = Henry's constant, and K1, K2, and Ky are equilibrium coefficients. The five unknowns in this system of five nonlinear equations are ct = total inorganic carbon, (HCO3] = bicarbonate, [co-) = = carbonate, [ht] = hydrogen ion, and [OH-] = hydroxyl ion. Notice how the partial pressure of CO2 shows up in Eqs. (8.6) and (8.9). Use these equations to compute the pH of rainwater given that Kg = 10-1.46, 10-6.3, K2 = 10-10.30 , and K = 10-14. Compare the results for 1958 when the Pcoz was 315 and for 2003 when it was 375 ppm. When selecting a numerical method for your computation, consider the following: You know with certainty that the pH of rain in pristine areas always falls between 2 and 12. . You also know that your measurement devices can only measure pH to two places of decimal precision. K = It is well documented that the atmospheric levels of several greenhouse gases have been increasing over the past 60 years. For example, Fig. 8.1 shows data for the partial pressure of carbon dioxide (CO2) collected at Mauna Loa, Hawaii, from 1958 through 2003. The trend in the data can be nicely fit with a quadratic polynomial (in Part Five, we will learn how to determine such polynomials), Pco2 = 0.011825(t 1980.5)2 + 1.356975 (t 1980.5) + 339 where Pco2 = the partial pressure of CO2 in the atmosphere (ppm). The data indicate that levels have increased during the period: from 315 to 376 ppm. Aside from global warming, greenhouse gases can also influence atmospheric chemistry. One question that we can address is how Page 210 the carbon dioxide trend is affecting the pH of rainwater. Outside of urban and industrial areas, it is well documented that carbon dioxide is the primary determinant of the pH of the rain. pH is the measure of the activity of hydrogen ions and, therefore, of acidity. For dilute aqueous solutions, it can be computed as (8.5) pH = -log10 [H] where [H+) is the molar concentration of hydrogen ions. The following five nonlinear system of equations govern the chemistry of rainwater, (8.6) [H+] HCO3] K1 = 10 KPco2 (8.7) [H+] [CO] K = (HCO3] (8.8) Kw = [HT] [OH-] (8.9) KPco2 = 106 + [HCO3] + [203] (8.10) 0 = [HCO3] +2 [co;-] + [OH-] - [h*] where Ku = Henry's constant, and K1, K2, and Ky are equilibrium coefficients. The five unknowns in this system of five nonlinear equations are ct = total inorganic carbon, (HCO3] = bicarbonate, [co-) = = carbonate, [ht] = hydrogen ion, and [OH-] = hydroxyl ion. Notice how the partial pressure of CO2 shows up in Eqs. (8.6) and (8.9). Use these equations to compute the pH of rainwater given that Kg = 10-1.46, 10-6.3, K2 = 10-10.30 , and K = 10-14. Compare the results for 1958 when the Pcoz was 315 and for 2003 when it was 375 ppm. When selecting a numerical method for your computation, consider the following: You know with certainty that the pH of rain in pristine areas always falls between 2 and 12. . You also know that your measurement devices can only measure pH to two places of decimal precision. K = It is well documented that the atmospheric levels of several greenhouse gases have been increasing over the past 60 years. For example, Fig. 8.1 shows data for the partial pressure of carbon dioxide (CO2) collected at Mauna Loa, Hawaii, from 1958 through 2003. The trend in the data can be nicely fit with a quadratic polynomial (in Part Five, we will learn how to determine such polynomials), Pco2 = 0.011825(t 1980.5)2 + 1.356975 (t 1980.5) + 339 where Pco2 = the partial pressure of CO2 in the atmosphere (ppm). The data indicate that levels have increased during the period: from 315 to 376 ppm








