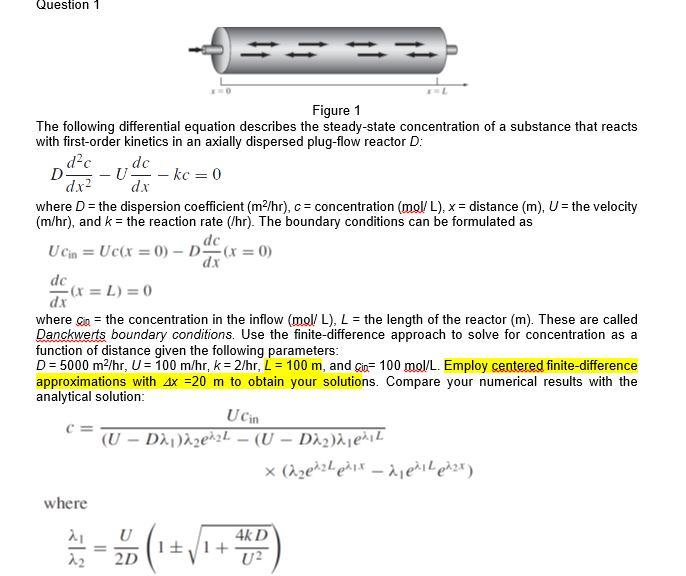
write a programme code by using matlab or C++ to solve this problem

Question 1 Figure 1 The following differential equation describes the steady-state concentration of a substance that reacts with first-order kinetics in an axially dispersed plug-flow reactor D: d-c .de D d.x? - kc = 0 dx where D = the dispersion coefficient (m2/hr), c= concentration (mol/L), x = distance (m), U = the velocity (m/hr), and k = the reaction rate (/hr). The boundary conditions can be formulated as dc U in = Ucx = 0)-D (x=0) dx dc F(x=L) = 0 dx where sia = the concentration in the inflow (mol/L), L = the length of the reactor (m). These are called Danckwerts boundary conditions. Use the finite-difference approach to solve for concentration as a function of distance given the following parameters: D = 5000 m2/hr, U = 100 m/hr, k = 2/hr, L = 100 m, and Gin= 100 mol/L. Employ centered finite-difference approximations with Ax =20 m to obtain your solutions. Compare your numerical results with the analytical solution: Ucin (U - D2e2L - (U - D22jen, x (Azenz Leal.- ajenilpa2x) where AL 11 U 2D 1+ 4KD 1 + U2 22 Question 1 Figure 1 The following differential equation describes the steady-state concentration of a substance that reacts with first-order kinetics in an axially dispersed plug-flow reactor D: d-c .de D d.x? - kc = 0 dx where D = the dispersion coefficient (m2/hr), c= concentration (mol/L), x = distance (m), U = the velocity (m/hr), and k = the reaction rate (/hr). The boundary conditions can be formulated as dc U in = Ucx = 0)-D (x=0) dx dc F(x=L) = 0 dx where sia = the concentration in the inflow (mol/L), L = the length of the reactor (m). These are called Danckwerts boundary conditions. Use the finite-difference approach to solve for concentration as a function of distance given the following parameters: D = 5000 m2/hr, U = 100 m/hr, k = 2/hr, L = 100 m, and Gin= 100 mol/L. Employ centered finite-difference approximations with Ax =20 m to obtain your solutions. Compare your numerical results with the analytical solution: Ucin (U - D2e2L - (U - D22jen, x (Azenz Leal.- ajenilpa2x) where AL 11 U 2D 1+ 4KD 1 + U2 22