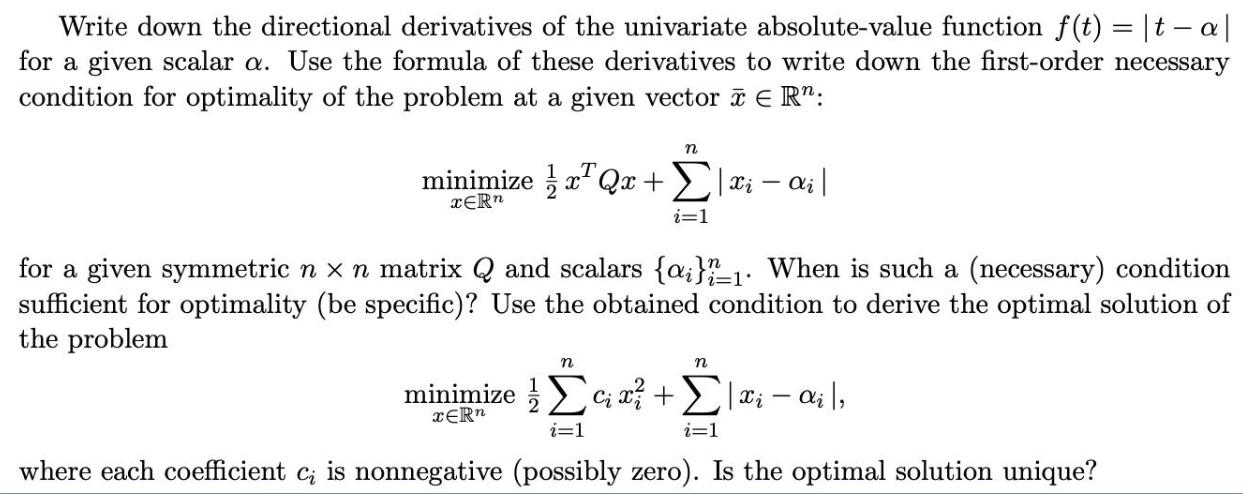
Write down the directional derivatives of the univariate absolute-value function f(t) = |t-a| for a given scalar a. Use the formula of these derivatives to write down the first-order necessary condition for optimality of the problem at a given vector x ER": minimize XERn n xQx+\xi = i\ i=1 for a given symmetric n n matrix Q and scalars {a} 1. When is such a (necessary) condition sufficient for optimality (be specific)? Use the obtained condition to derive the optimal solution of the problem n n minimize ca + ai, XERn i=1 i=1 where each coefficient c; is nonnegative (possibly zero). Is the optimal solution unique?