WRITE THE CODE IN C++

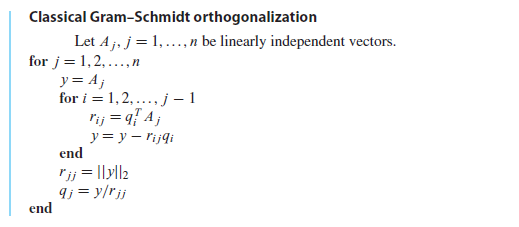
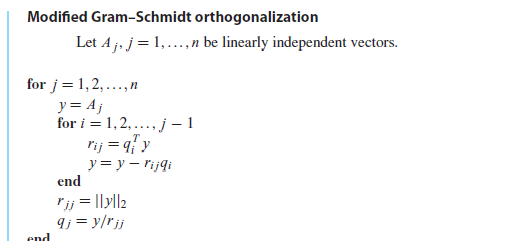
Write a code that implements both the classical and the modified GramSchmidt orthogonalization to find the reduced QR factorization of a given matrix A. Your code should detect if the columns are linearly dependent and abort with an appropriate message.

To detect linear dependence of the column vectors, it's sufficient to check for zero before normalizing vectors v_j in the Classical Gramm-Schmidt (CGS), v_i in the Modified Gramm-Schmidt (MGS) and v_k in the Householder (H) methods, respectively. At least for CGS and MGS it should be clear that zero length of these vectors means that they are in the span of all previous q vectors. Recall that for each j=1:n, span{a₁,..,a_j} = span{q₁,...,q_j}. So in short, in CGS you have to stop the routine if r_jj=0; likewise in MGS if r_ii=0; in H if norm(v_k)=0.

Classical Gram-Schmidt orthogonalization Let Aj, j = l, .. ., n be linearly independent vectors. for j=1,2, ,n for i = 1 , 2. ,j-1 end end Modified Gram-Schmidt orthogonalization Let AN = 1, , n be linearly independent vectors. for j =1,2, ,n for i = 1 , 2. . J-1 y = y-rijq end Classical Gram-Schmidt orthogonalization Let Aj, j = l, .. ., n be linearly independent vectors. for j=1,2, ,n for i = 1 , 2. ,j-1 end end Modified Gram-Schmidt orthogonalization Let AN = 1, , n be linearly independent vectors. for j =1,2, ,n for i = 1 , 2. . J-1 y = y-rijq end