X2
Kindly help
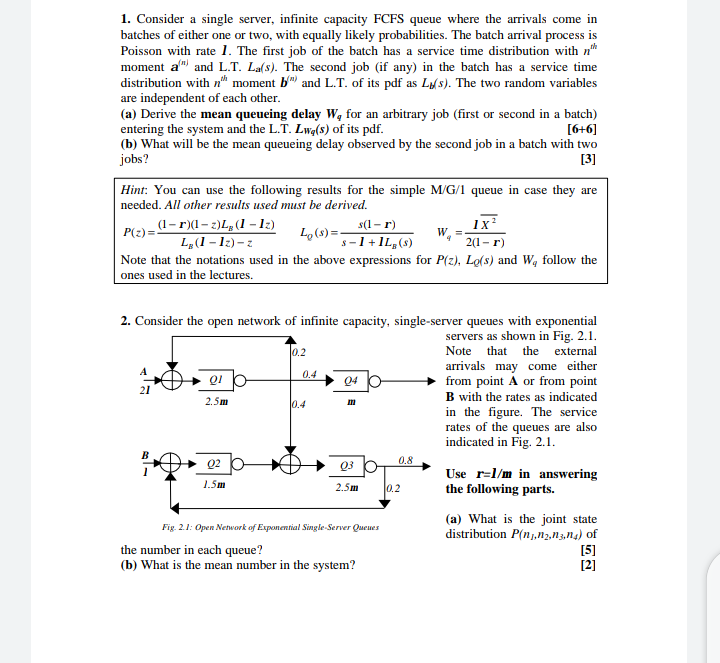
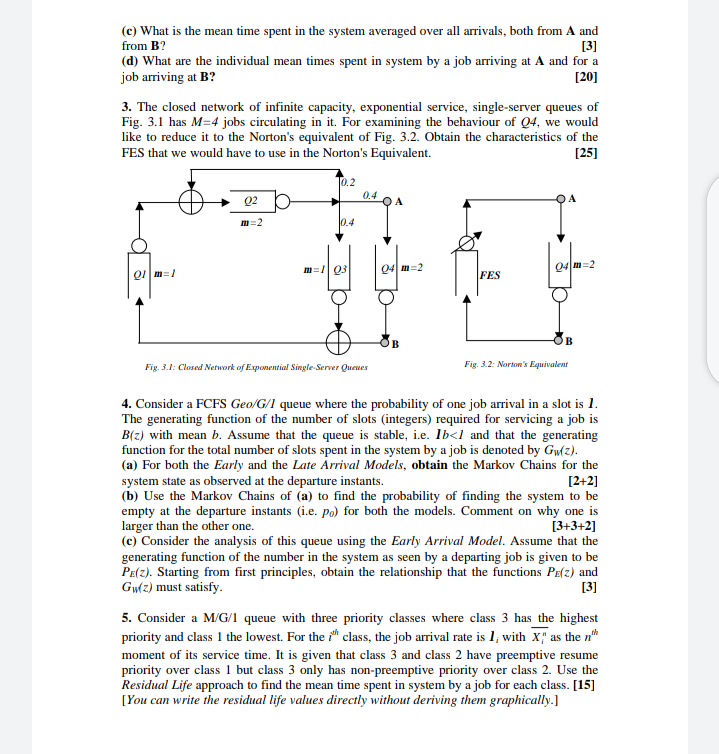
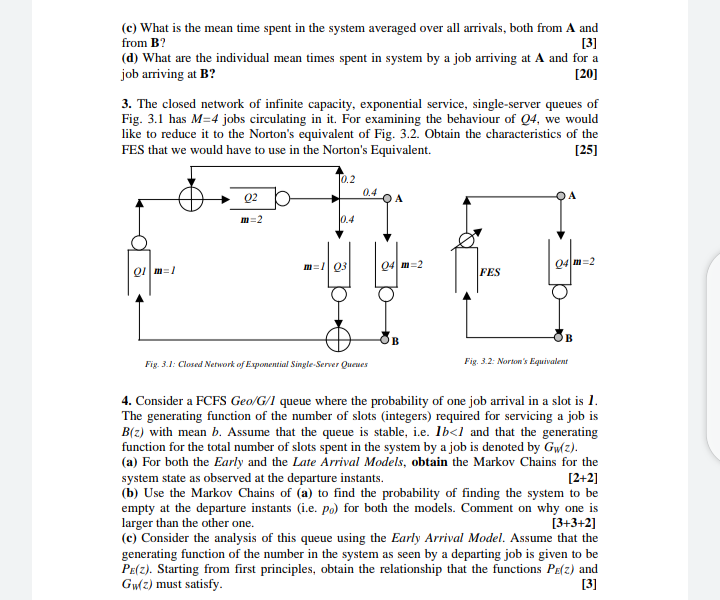
1. Consider a single server, infinite capacity FCFS queue where the arrivals come in batches of either one or two, with equally likely probabilities. The batch arrival process is Poisson with rate 1. The first job of the batch has a service time distribution with n" moment a" and L.T. La(s). The second job (if any) in the batch has a service time distribution with a" moment b" and L.T. of its pdf as Ly(s). The two random variables are independent of each other. (a) Derive the mean queueing delay W, for an arbitrary job (first or second in a batch) entering the system and the L.T. Lwq(s) of its pdf. [6+6] (b) What will be the mean queueing delay observed by the second job in a batch with two jobs? [3] Hint: You can use the following results for the simple M/G/1 queue in case they are needed. All other results used must be derived. P(2)= (-r)(1-2)L, (1 - 12) Lo (s) = - s(1 - r) IX' W L, (1 - 12) - 2 8- 1 +1L,($) 201- r) Note that the notations used in the above expressions for P(z), Lo(s) and W, follow the ones used in the lectures. 2. Consider the open network of infinite capacity, single-server queues with exponential servers as shown in Fig. 2.1. 0.2 Note that the external arrivals may come either 0.4 04 O from point A or from point 2.5m 104 B with the rates as indicated in the figure. The service rates of the queues are also indicated in Fig. 2.1. 23 0.8 Use r=1/m in answering 1.5mm 2.5m 0. 2 the following parts. Fig. 2.1: Open Network of Exponential Single-Server Queues (a) What is the joint state distribution P(ny,nz,na,n,) of the number in each queue? [5] (b) What is the mean number in the system? [2](c) What is the mean time spent in the system averaged over all arrivals, both from A and from B? [3] (d) What are the individual mean times spent in system by a job arriving at A and for a job arriving at B? [20] 3. The closed network of infinite capacity, exponential service, single-server queues of Fig. 3.1 has M=4 jobs circulating in it. For examining the behaviour of 04, we would like to reduce it to the Norton's equivalent of Fig. 3.2. Obtain the characteristics of the FES that we would have to use in the Norton's Equivalent. [25] 0.2 02 OA 04 m=2 04 m=2 Or m= 1 FES Fig. 3.1: Closed Network of Exponential Single-Server Queues Fig. 3.2: Norton's Equivalent 4. Consider a FCFS Geo/G/I queue where the probability of one job arrival in a slot is 1. The generating function of the number of slots (integers) required for servicing a job is B(z) with mean b. Assume that the queue is stable, i.e. 1b and that the generating function for the total number of slots spent in the system by a job is denoted by Gwz). (a) For both the Early and the Late Arrival Models, obtain the Markov Chains for the system state as observed at the departure instants. [2+2] (b) Use the Markov Chains of (a) to find the probability of finding the system to be empty at the departure instants (i.e. po) for both the models. Comment on why one is larger than the other one. [3+3+2] (c) Consider the analysis of this queue using the Early Arrival Model. Assume that the generating function of the number in the system as seen by a departing job is given to be PE(z). Starting from first principles, obtain the relationship that the functions Ps(z) and Gw(z) must satisfy. [3] 5. Consider a M/G/1 queue with three priority classes where class 3 has the highest priority and class I the lowest. For the /" class, the job arrival rate is ], with X, as the n" moment of its service time. It is given that class 3 and class 2 have preemptive resume priority over class 1 but class 3 only has non-preemptive priority over class 2. Use the Residual Life approach to find the mean time spent in system by a job for each class. [15] [ You can write the residual life values directly without deriving them graphically.](c) What is the mean time spent in the system averaged over all arrivals, both from A and from B? [3] (d) What are the individual mean times spent in system by a job arriving at A and for a job arriving at B? [20] 3. The closed network of infinite capacity, exponential service, single-server queues of Fig. 3.1 has M=4 jobs circulating in it. For examining the behaviour of 04, we would like to reduce it to the Norton's equivalent of Fig. 3.2. Obtain the characteristics of the FES that we would have to use in the Norton's Equivalent. [25] 0.2 02 04 0 A OA 10.4 Q/ m= 1 m=1 03 04 m=2 04 m=2 FES OB Fig. 3.1: Closed Network of Exponential Single-Server Queues Fig. 3.2: Norton's Equivalent 4. Consider a FCFS Geo/G// queue where the probability of one job arrival in a slot is 1. The generating function of the number of slots (integers) required for servicing a job is B(z) with mean b. Assume that the queue is stable, i.e. 1b and that the generating function for the total number of slots spent in the system by a job is denoted by Gwz). (a) For both the Early and the Late Arrival Models, obtain the Markov Chains for the system state as observed at the departure instants. [2+2] (b) Use the Markov Chains of (a) to find the probability of finding the system to be empty at the departure instants (i.e. po) for both the models. Comment on why one is larger than the other one. [3+3+2] (c) Consider the analysis of this queue using the Early Arrival Model. Assume that the generating function of the number in the system as seen by a departing job is given to be PE(z). Starting from first principles, obtain the relationship that the functions PE(z) and Gw(z) must satisfy. [3]