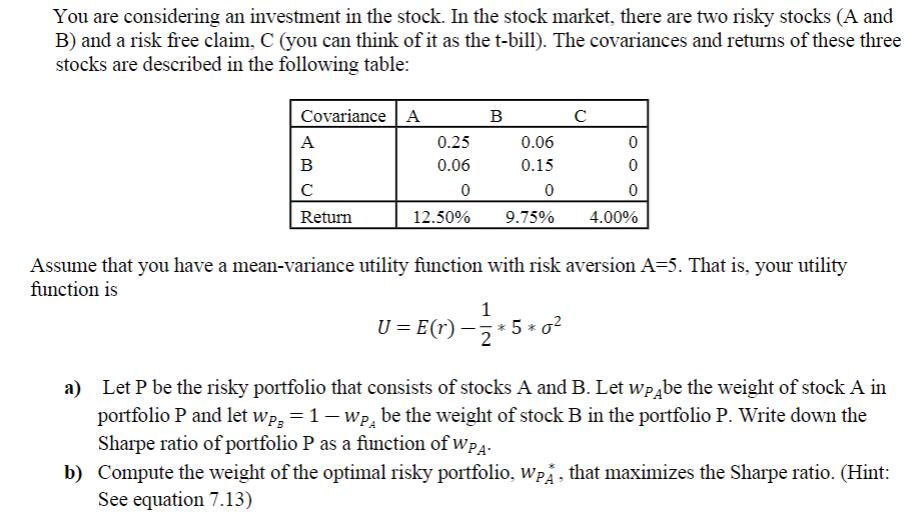
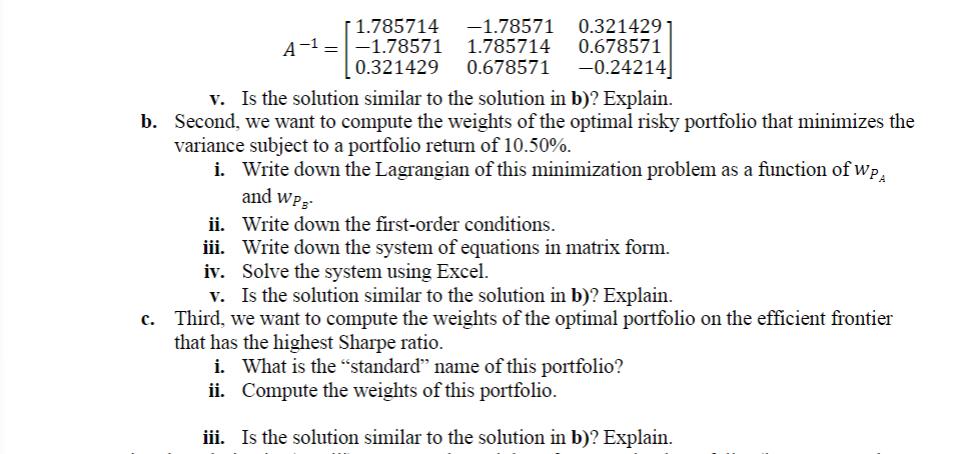
You are considering an investment in the stock. In the stock market, there are two risky stocks (A and B) and a risk free claim, C (you can think of it as the t-bill). The covariances and returns of these three stocks are described in the following table: Covariance A A ABC C Return 0.25 0.06 0 12.50% B U = E(r) - 0.06 0.15 0 9.75% 1 2 Assume that you have a mean-variance utility function with risk aversion A=5. That is, your utility function is C 5 * 0 0 0 0 4.00% a) Let P be the risky portfolio that consists of stocks A and B. Let wp be the weight of stock A in portfolio P and let Wp = 1 - WP. be the weight of stock B in the portfolio P. Write down the Sharpe ratio of portfolio P as a function of WPA- b) Compute the weight of the optimal risky portfolio, w, that maximizes the Sharpe ratio. (Hint: See equation 7.13) c) However, instead of maximizing the Sharpe ratio directly, you decide to use the Markowitz Portfolio Optimization Model. In the next couple of questions, we will compute the optimal risky portfolio using the Markowitz procedure. As before, let wo be the weight of stock A in portfolio P and let wp = 1 w be the weight of stock B in the portfolio P. a. First, we want to find the weights of the optimal risky portfolio that minimizes the variance. i. Write the down the Lagrangian of this minimization problem as a function of Wp and WP g ii. Write down the first-order conditions. iii. Write down the system of equations in matrix form. iv. Solve the system. In this question you are expected to solve the system in matrix form by hand (I urge you to do it!). Please include (only) the solution. (Use Excel to double check your answer). Hint: In this case, the matrix A- is: A- [1.785714 -1.78571 0.3214291 -1.78571 1.785714 0.678571 0.321429 0.678571 -0.24214] v. Is the solution similar to the solution in b)? Explain. b. Second, we want to compute the weights of the optimal risky portfolio that minimizes the variance subject to a portfolio return of 10.50%. i. Write down the Lagrangian of this minimization problem as a function of WPA and WP g ii. Write down the first-order conditions. iii. Write down the system of equations in matrix form. iv. Solve the system using Excel. v. Is the solution similar to the solution in b)? Explain. c. Third, we want to compute the weights of the optimal portfolio on the efficient frontier that has the highest Sharpe ratio. i. What is the "standard" name of this portfolio? ii. Compute the weights of this portfolio. iii. Is the solution similar to the solution in b)? Explain.