You bought a 1 kg solid gold statue from a merchant in Italy while you are on vacation. When you get home, you decided to test if this statue is real gold or not. After submerging the gold statue in a large water container, you will measure the volume of displaced water. What is the expected volume if the statue is made of pure gold? density of gold is 19300kg/m^3 Trying to figure it out with the notes that I have. I don't understand where the numbers should go. Thank you.

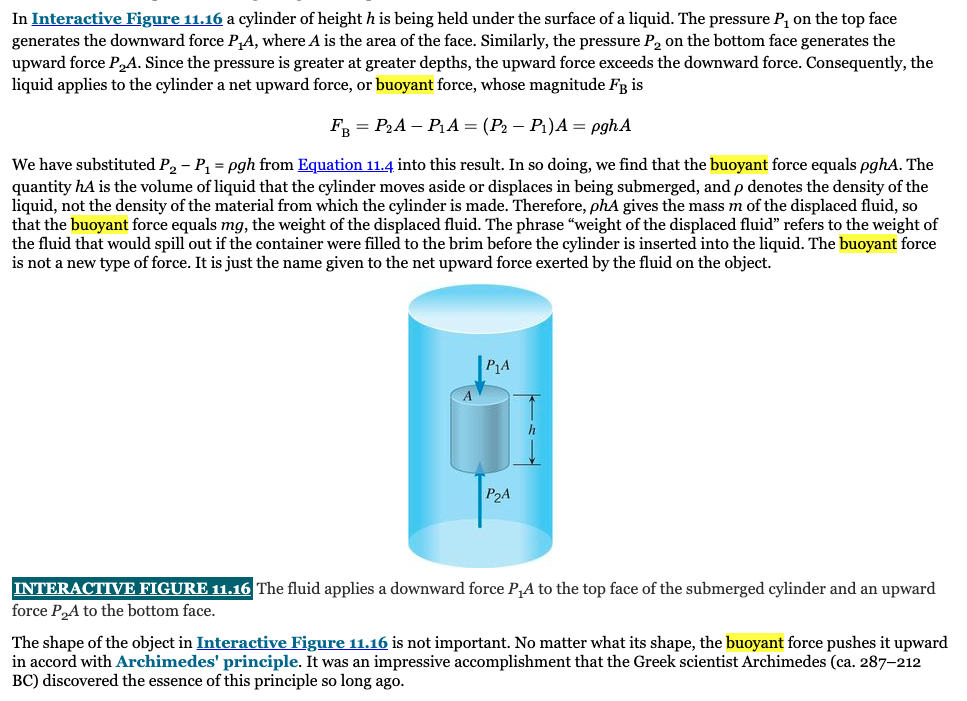
Magnitude of Buoyant Force = Weight of Displaced Fluid Bernoulli's Equation and Its Application When fluids are in motion, they move with a variety of options. You may have observed that water flows calmly in a shallow stream and violently in a steep valley. The air blows very gently sometimes and vigorously with great speed at other times. In order to characterize the type of fluids, compressible/incompressible and viscousonviscous categories are used. When the density of a fluid is almost constant, the fluid is said to be incompressible. Luckily, most liquids are incompressible, but all gases are not. When fluid like honey does not flow easily, it is a viscous fluid. On the other hand, water flows very easily because its viscosity is low. Incompressible and nonviscous fluids are called ideal fluids; this is great to describe the motion of fluids with mathematical equations. For steady flow, Bernoulli studied the behavior of ideal fluids. Its result is in his equation: P + 1/2 pv + pgy = constant. In this equation, y is the elevation at any point, and v is the fluid speed. If the flow speed is not changing, the above equation goes back to the pressure equation when water is at rest. If the flow is horizontal, that is, there is no elevation between two points, the pressure is related to the speed. The higher fluid pressure makes the slow-moving flow and vice versa. In addition, when the volume flow rate Av = constant, if the cross-sectional area of a tube is large, the fluid speed is small and vice versa. With these fluid equations, we can describe the motion of liquids in a plumbing system, the speed changes of oil in a pipe, and even the dynamics of an airplane wing.In Interactive Figure 11.16 a cylinder of height h is being held under the surface of a liquid. The pressure P, on the top face generates the downward force PIA, where A is the area of the face. Similarly, the pressure P, on the bottom face generates the upward force PA. Since the pressure is greater at greater depths, the upward force exceeds the downward force. Consequently, the liquid applies to the cylinder a net upward force, or buoyant force, whose magnitude FB is FB = P2A - PIA = (P2 - PI)A = pghA We have substituted P2 - P1 = pgh from Equation 11.4 into this result. In so doing, we find that the buoyant force equals pghA. The quantity hA is the volume of liquid that the cylinder moves aside or displaces in being submerged, and p denotes the density of the liquid, not the density of the material from which the cylinder is made. Therefore, phA gives the mass m of the displaced fluid, so that the buoyant force equals my, the weight of the displaced fluid. The phrase "weight of the displaced fluid" refers to the weight of the fluid that would spill out if the container were filled to the brim before the cylinder is inserted into the liquid. The buoyant force is not a new type of force. It is just the name given to the net upward force exerted by the fluid on the object. |PIA P2A INTERACTIVE FIGURE 11.16 The fluid applies a downward force ,A to the top face of the submerged cylinder and an upward force PA to the bottom face. The shape of the object in Interactive Figure 11.16 is not important. No matter what its shape, the buoyant force pushes it upward in accord with Archimedes' principle. It was an impressive accomplishment that the Greek scientist Archimedes (ca. 287-212 BC) discovered the essence of this principle so long ago