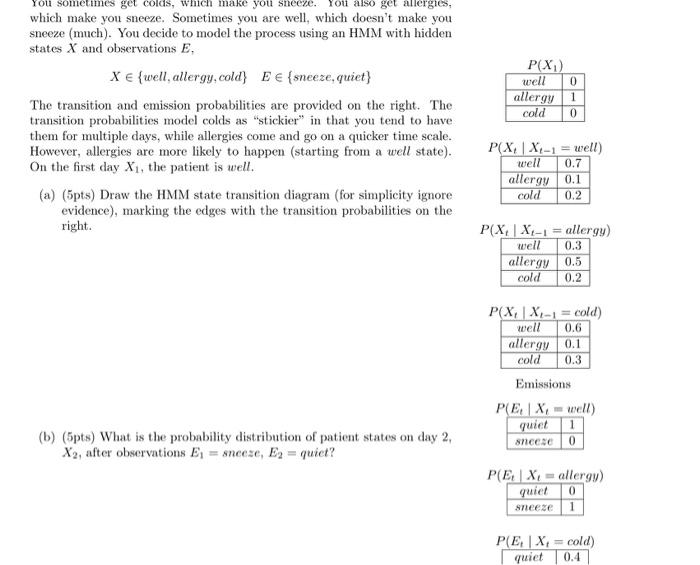
You sometimes get colds, which make you knceze. You also get allergies, which make you sneeze. Sometimes you are well, which doesn't make you sneeze (much). You decide to model the process using an HMM with hidden states X and observations E. X {well, allergy, cold) E (sneeze,quiet) The transition and emission probabilities are provided on the right. The transition probabilities model colds as "stickier" in that you tend to have them for multiple days, while allergies come and go on a quicker time scale. However, allergies are more likely to happen (starting from a well state). On the first day Xy, the patient is well. (a) (5pts) Draw the HMM state transition diagram (for simplicity ignore evidence), marking the edges with the transition probabilities on the right. PCX1) well 0 allergy 1 cold 0 P(X. X-1 = well) well 0.7 allergy 0.1 cold 0.2 P(X.X-1 = allergy) well 0.3 allergy 0.5 cold 0.2 P(X, X:-1 = cold) well 0.6 allergy 0.1 cold 0.3 Emissions (b) (5pts) What is the probability distribution of patient states on day 2, Xy, after observations Ej = sneeze, Es = quiet? P( EX: = well) quiet 1 sneeze 0 P( EX: = allergy) quiet 0 sneese 1 P( EX cold) quiet Sneeze 0.6 0.4 You sometimes get colds, which make you sreeze. You also get allergies. which make you snecze. Sometimes you are well, which doesn't make you sneeze (much). You decide to model the process using an HMM with hidden states X and observations E. X {well, allergy, cold} E {sneeze, quiet) The transition and emission probabilities are provided on the right. The transition probabilities model colds as "stickier" in that you tend to have them for multiple days, while allergies come and go on a quicker time scale. However, allergies are more likely to happen (starting from a well state). On the first day X, the patient is well. (a) (5pts) Draw the HMM state transition diagram (for simplicity ignore evidence), marking the edges with the transition probabilities on the right. P(X) 0 allergy 1 cold 0 well P(X, X-1 = well) well 0.7 allergy 0.1 cold 0.2 P(X, X:-1 = allergy) well 0.3 allergy 0.5 cold 0.2 P(X | X-1 = cold) well 0.6 allergy 0.1 cold 0.3 Emissions PE | Xe = tell) quiet 1 0 (b) (5pts) What is the probability distribution of patient states on day 2. X2, after observations Es = sneeze, E2 = quiet? ancese P( EX, -allergy) quiet 0 sneeze 1 P( EXi = cold) quiet 0.4 0.6 ancese (c) (5pts) What is the probability distribution of the patient states on day 3, X3, after observing E1 = sneeze, E. = quiet, Es = sneeze. P(X1) well 0 allergy 1 cold 0 You sometimes get colds, which make you sneeze. You also get allergies, which make you sneeze. Sometimes you are well, which doesn't make you sneeze (much). You decide to model the process using an HMM with hidden states X and observations E. X {well, allergy, cold} E (sneeze, quiet} The transition and emission probabilities are provided on the right. The transition probabilities model colds as "stickier" in that you tend to have them for multiple days, while allergies come and go on a quicker time scale. However, allergies are more likely to happen (starting from a well state). On the first day X1, the patient is well. (a) (5pts) Draw the HMM state transition diagram (for simplicity ignore evidence), marking the edges with the transition probabilities on the right. PCX, X, -1 = well) well 0.7 allergy 0.1 cold 0.2 P(X:X-= allergy) well 0.3 allergy 0.5 cold 0.2 PCX, X:-1 = cold) well 0.6 allergy 0.1 0.3 cold Emissions P( EX, well) quiet 1 ancese 0 (b) (5pts) What is the probability distribution of patient states on day 2, X2, after observations E = sneeze, Es = quiet? PEX-allerg) 0 1 sneeze P(E | X, =cold) quiet 0.4 P(X, X-1 = cold) well 0.6 allergy 0.1 cold 0.3 Emissions P(Et |X= well) quiet 1 sneeze 0 (b) (5pts) What is the probability distribution of patient states on day 2, Xy, after observations E1 = sneeze, Ex = quiet? P( EX, = allergy) quiet 0 1 sneeze P( EX, =cold) quict 0.4 sneeze 0.6 (c) (5pts) What is the probability distribution of the patient states on day 3, X3, after observing E = sneeze, E, = quiet, Es = sneeze. You sometimes get colds, which make you knceze. You also get allergies, which make you sneeze. Sometimes you are well, which doesn't make you sneeze (much). You decide to model the process using an HMM with hidden states X and observations E. X {well, allergy, cold) E (sneeze,quiet) The transition and emission probabilities are provided on the right. The transition probabilities model colds as "stickier" in that you tend to have them for multiple days, while allergies come and go on a quicker time scale. However, allergies are more likely to happen (starting from a well state). On the first day Xy, the patient is well. (a) (5pts) Draw the HMM state transition diagram (for simplicity ignore evidence), marking the edges with the transition probabilities on the right. PCX1) well 0 allergy 1 cold 0 P(X. X-1 = well) well 0.7 allergy 0.1 cold 0.2 P(X.X-1 = allergy) well 0.3 allergy 0.5 cold 0.2 P(X, X:-1 = cold) well 0.6 allergy 0.1 cold 0.3 Emissions (b) (5pts) What is the probability distribution of patient states on day 2, Xy, after observations Ej = sneeze, Es = quiet? P( EX: = well) quiet 1 sneeze 0 P( EX: = allergy) quiet 0 sneese 1 P( EX cold) quiet Sneeze 0.6 0.4 You sometimes get colds, which make you sreeze. You also get allergies. which make you snecze. Sometimes you are well, which doesn't make you sneeze (much). You decide to model the process using an HMM with hidden states X and observations E. X {well, allergy, cold} E {sneeze, quiet) The transition and emission probabilities are provided on the right. The transition probabilities model colds as "stickier" in that you tend to have them for multiple days, while allergies come and go on a quicker time scale. However, allergies are more likely to happen (starting from a well state). On the first day X, the patient is well. (a) (5pts) Draw the HMM state transition diagram (for simplicity ignore evidence), marking the edges with the transition probabilities on the right. P(X) 0 allergy 1 cold 0 well P(X, X-1 = well) well 0.7 allergy 0.1 cold 0.2 P(X, X:-1 = allergy) well 0.3 allergy 0.5 cold 0.2 P(X | X-1 = cold) well 0.6 allergy 0.1 cold 0.3 Emissions PE | Xe = tell) quiet 1 0 (b) (5pts) What is the probability distribution of patient states on day 2. X2, after observations Es = sneeze, E2 = quiet? ancese P( EX, -allergy) quiet 0 sneeze 1 P( EXi = cold) quiet 0.4 0.6 ancese (c) (5pts) What is the probability distribution of the patient states on day 3, X3, after observing E1 = sneeze, E. = quiet, Es = sneeze. P(X1) well 0 allergy 1 cold 0 You sometimes get colds, which make you sneeze. You also get allergies, which make you sneeze. Sometimes you are well, which doesn't make you sneeze (much). You decide to model the process using an HMM with hidden states X and observations E. X {well, allergy, cold} E (sneeze, quiet} The transition and emission probabilities are provided on the right. The transition probabilities model colds as "stickier" in that you tend to have them for multiple days, while allergies come and go on a quicker time scale. However, allergies are more likely to happen (starting from a well state). On the first day X1, the patient is well. (a) (5pts) Draw the HMM state transition diagram (for simplicity ignore evidence), marking the edges with the transition probabilities on the right. PCX, X, -1 = well) well 0.7 allergy 0.1 cold 0.2 P(X:X-= allergy) well 0.3 allergy 0.5 cold 0.2 PCX, X:-1 = cold) well 0.6 allergy 0.1 0.3 cold Emissions P( EX, well) quiet 1 ancese 0 (b) (5pts) What is the probability distribution of patient states on day 2, X2, after observations E = sneeze, Es = quiet? PEX-allerg) 0 1 sneeze P(E | X, =cold) quiet 0.4 P(X, X-1 = cold) well 0.6 allergy 0.1 cold 0.3 Emissions P(Et |X= well) quiet 1 sneeze 0 (b) (5pts) What is the probability distribution of patient states on day 2, Xy, after observations E1 = sneeze, Ex = quiet? P( EX, = allergy) quiet 0 1 sneeze P( EX, =cold) quict 0.4 sneeze 0.6 (c) (5pts) What is the probability distribution of the patient states on day 3, X3, after observing E = sneeze, E, = quiet, Es = sneeze