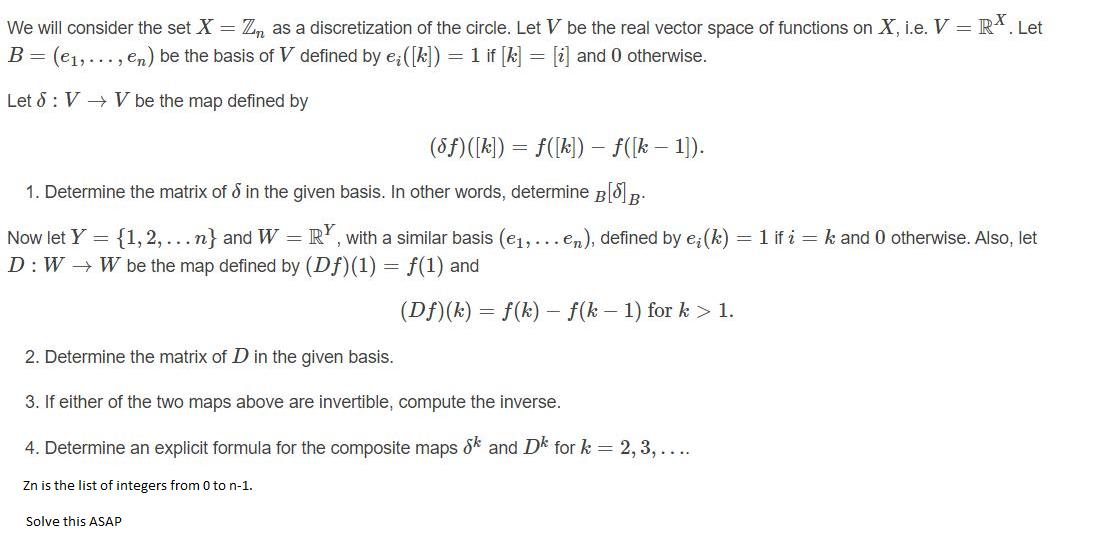
We will consider the set X = Z, as a discretization of the circle. Let V be the real vector space of functions on X, i.e. V = RX. Let B = (e,..., en) be the basis of V defined by e; ([k]) = 1 if [k] = [i] and 0 otherwise. Let 8: VV be the map defined by (Sf) ([k]) = f([k]) ([k 1]). - 1. Determine the matrix of 8 in the given basis. In other words, determine B[] B. Now let y = {1,2,... n} and W = RY, with a similar basis (e,... en), defined by e; (k) = 1 if i = k and 0 otherwise. Also, let D: W W be the map defined by (Df)(1) = f(1) and (Df)(k) = f(k) - f(k-1) for k> 1. 2. Determine the matrix of D in the given basis. 3. If either of the two maps above are invertible, compute the inverse. 4. Determine an explicit formula for the composite maps 8k and Dk for k= 2, 3,.... Zn is the list of integers from 0 to n-1. Solve this ASAP