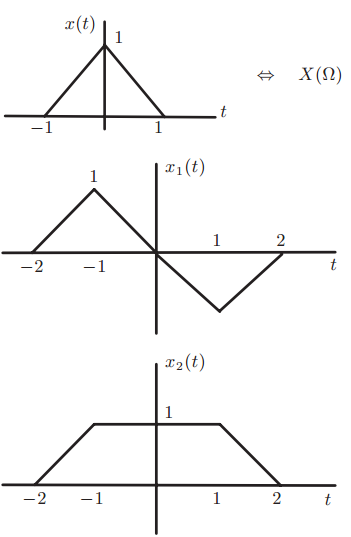
If the Fourier transform of the pulse x(t) given in Figure 5.14 is X(Ω) (do not need to compute it)

(a) Using the properties of the Fourier transform (no integration needed) obtain the Fourier transforms of the signals x_i(t), i = 1, 2, shown in Figure 5.14 in terms of X(Ω).

(b) Consider the modulated signal x₃(t) = x(t) cos(Ï€ t). Plot x₃(t) and express its Fourier transform X₃(Ω) in terms of X(Ω).

(c) If y(t) = x(t ˆ’ 1) find Y(0).

Figure 5.14:

Transcribed Image Text:

r(t) X (2) T1(t) -2 X2(t) -2 -1