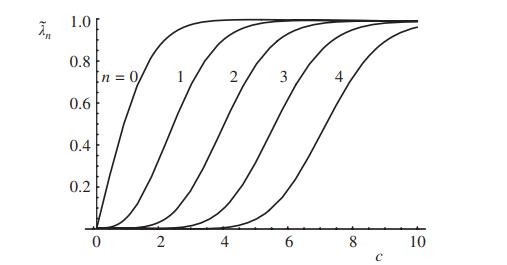
Examination of Fig. 6.5 shows that a relatively abrupt threshold in values of \(\tilde{\lambda}_{n}\) occurs as \(n\) is varied. In particular, as a rough approximation,

\[ \tilde{\lambda}_{n} \approx \begin{cases}1 & nn_{\text {crit }}\end{cases} \]<_>

where \(n_{\text {crit }}=2 c / \pi\). Show that this approximate distribution of \(\tilde{\lambda}_{n}\) leads to a gamma probability density function for the integrated intensity \(W\). Compare the number \(n_{\text {crit }}\) with the parameter \(\mathcal{M}\).

Transcribed Image Text:

In 1.0 0.8 n = 0 2 3 0.6 0.4 0.2 0 2 4 6 8 + C 10