The kurtosis of a variable represents a measure of how the distribution of a variable is peaked (in other words, how "sharp" the data set appears when you consider its distribution). The kurtosis can be used to gauge whether the distribution of a variable differs from the normal distribution. For instance, the closer the kurtosis value is to 0 , the more normally distributed the variable is; the closer the kurtosis value is to 1 , the sharper the peak of the distribution; and a negative kurtosis value represents more of a flat distribution. The formula for kurtosis is as follows:

\[\text { Kurtosis }=\left[\frac{n(n+1)}{(n-1)(n-2)(n-3)}ight] \cdot\sum_{i=1}^{n}\left(\frac{x_{i}-\bar{x}}{s}ight)^{4}-\frac{3(n-1)^{2}}{(n-2)(n-3)}\]

where \(n\) is the sample size, \(x_{i}\) are the individual observations, \(\bar{x}\) is the sample mean, and \(s\) is the sample standard deviation for the given data.

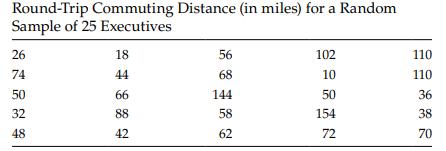
a. For the round-trip commuting distance data set in Table 2.1, draw a histogram and calculate the value of the kurtosis statistic.

b. Does the measure of kurtosis make sense for the shape of the distribution for the commuting distance data set in Table 2.1?

Table 2.1

Transcribed Image Text:

Round-Trip Commuting Distance (in miles) for a Random Sample of 25 Executives 26 18 56 102 110 74 44 68 10 110 50 66 144 50 36 32 88 58 154 38 48 42 62 72 70