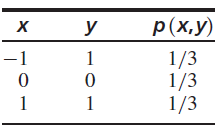
Here are two random variables that are uncorrelated but not independent Let X and Y have the following joint probability mass function a Use the definition of independence on page 141 to show that X and Y are not independent (in fact Y X , so Y is actually a function of X) b Show that X and Y are u...

a PX 1 13 PY 1 23 PX 1 and Y 1 13 6 ...

Here are two random variables that are uncorrelated but not independent. Let X and Y have the

Question:

Here are two random variables that are uncorrelated but not independent. Let X and Y have the following joint probability mass function:

p (х,у) х y -1 1 1/3 1/3 1/3

a. Use the definition of independence on page 141 to show that X and Y are not independent (in fact
Y = |X|, so Y is actually a function of X).

b. Show that X and Y are uncorrelated.

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

Statistics For Engineers And Scientists

ISBN: 9780073401331

4th Edition

Authors: William Navidi

See More Books

Question Posted: Nov 02, 2018 09:18 AM

See More Questions

Find an example (other than the one given in Example 5.15) of two random variables that are uncorrelated but not independent.
Suppose that X and Y are two random variables, which may be dependent, and Var(X) = Var(Y). Assuming that 0 < Var(X + Y) < and 0 < Var(X Y) < , show that the random variables X + Y and X Y are...
Let D 1 and D 2 be the outcomes of two dice rolls. Let X = D 1 + D 2 be the sum of the two numbers rolled. Let Y = D 1 D 2 be their difference. Show that X and Y are uncorrelated, but not...
Show that 1. Every differentiable concave function is pseudoconcave. 2. Every pseudoconcave function is quasiconcave
Are you a member of the "thumb generation"? Can you work the keyboard of your smartphone faster than most people can speak? The term thumb generation was coined in South Korea and Japan and is...
Required information The Foundational 15 (Static) (LO4-1, LO4-2, LO4-3, LO4-4, LO4-5) [The following information applies to the questions displayed below.] Clopack Company manufactures one product...
How are risk-adjusted discount rates used to incorporate project risk into the capital budgeting decision process? AppendixLO1
A local drama company proposes a new neighborhood theater in San Francisco. Before approving the building permit, the city planner completes a study of the theaters impact on the surrounding...
Can i get some help on these two please my work ak Sachs Brands's defined benefit pension plan specifies annual retirement benefits equal to 1.6% * service years * final year's salary, payable at the...
If an assets expected return does not plot on the line in question 2 above, what does that imply about its price?
Refer to Exercise 23. Assume that the probabilities that each of the three scenarios occurs are P(A) = 0.20, P(B) = 0.30, and P(C) = 0.50. a. Find the probability that scenario A occurs and that the...
Refer to Exercise 21. Is it possible for the probability that both gauges fail to be greater than 0.01? Explain. Refer to Exercise 21. Nuclear power plants have redundant components in important...
When the trend is the most important item to communicate, which of the following statements is true? a. Bar charts are preferable to line charts. b. Line charts are preferable to bar charts. c. Bar...
Case study: Sun City - improving operations performance to enhance guest experience 1. Describe how Sun City implements the five operations performance objectives or principles. 2. Using your...
What recommendations do you have to increase the likelihood of success? E.g., how would you reduce the likelihood of having to go back to A4? How would you reduce the impact of having to go back to...
Problem 4 An electrically heated, square plate (0.4mx 0.4 mx0.005 m) is suspended in air of temperature Too = 20C. Find the electrical power needed to maintain the plate at T=95C if the plate is (a)...
Number of units Unit Cost Sales Beginning inventory 800 $50 Purchased 600 $52 Sold 400 $80 Sold 350 $90 Ending inventory 650 In the table below, calculate the dollar value for the period for each of...
10. Dr. D went to MGM Springfield casino while the class was taking their midterm exam. He played a Konami machine entitled 88 Fortunes. A slot attendant accidently left the slot manual next to the...
Solve a. b. c. d.|3x 5| = x + 1 e. x +|x + 4 |= 8 f. 8 |1 2x| = x 2x + 1 x-21 = 5
How has the globalization of firms affected the diversity of their employees? Why has increased diversity put an additional burden on accounting systems?
The article "Advances in Oxygen Equivalence Equations for Predicting the Properties of Titanium Welds" (D. Harwig, W. Ittiwattana, and H. Castner, The Welding Journal, 2001:126s-136s) reports an...
The article "Advances in Oxygen Equivalence Equations for Predicting the Properties of Titanium Welds" (D. Harwig, W. Ittiwattana, and H. Castner, The Welding Journal, 2001:126s-136s) reports an...
The data presented in the following table give the tensile strength in psi (y) of paper as a function of the percentage of hardwood content (x). a. Fit polynomial models of degrees 1, 2, and so on to...
Harper, Inc, acquires 40 percent of the outstanding voting stock of Kinman Company on January 1, 2020, for $316,100 in cash. The book value of Kinman's net assets on that date was $610.000, although...
Need a help for this! The following information is obtained from the records of Kaiser Company: On January 1, 2017 the following machines were acquired for cash: Production machines costs $ 6,000...
Book 51,500 Hint rint rences Raw Materials Inventory Debit Credit Beginning 10,100 Purchases 45,500 Available for use 55,600 DM used Ending 4,100 Work in Process Inventory Debit Credit Beginning...

Here are two random variables that are uncorrelated but not independent. Let X and Y have the

Question:

p (х,у) х y -1 1 1/3 1/3 1/3

Step by Step Answer:

a PX 1 13 PY 1 23 PX 1 and Y 1 13 6 ...View the full answer

Statistics For Engineers And Scientists

Students also viewed these Mathematics questions