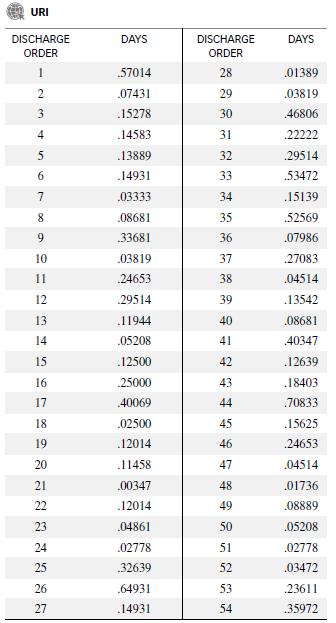
In Quality Engineering (Vol. 25, 2013), statisticians at Minitab, Inc., applied control chart methodology to monitor the time between discharges of male patients with hospital-acquired urinary tract infections (URI). The data (days between discharges) for n = 54 consecutive URI discharges at a large hospital system are shown in the table.

a. Construct a control chart for the variable, time between discharges (in days). Does the process appear to be in control?

b. The statisticians demonstrated that time between discharges follows an exponential distribution rather than a normal distribution. Consequently, the control limits on the control chart, part a, will not yield the rare event probabilities that form the basis of control chart methodology. They derived the control limits for an exponential random variable with mean θ as follows:

LCL = .001351(θ), Center line = θln(2), UCL = 6.60773(θ)

Show that for an exponential random variable Y, P(Y < LCL) = P(Y > UCL) ≈ .001 and P(Y > Center line) = .5.

c. Use the control limits given in part b and the fact that the mean time between discharges is .21 days to construct a control chart for the time between urinary tract infections. Does the process appear to be in control now?

Transcribed Image Text:

URI DISCHARGE DAYS DISCHARGE DAYS ORDER ORDER 57014 28 .01389 .07431 29 .03819 3 15278 30 46806 4 .14583 31 22222 .13889 32 29514 6. .14931 33 .53472 03333 34 .15139 8. .08681 35 .52569 9. 33681 36 .07986 10 .03819 37 .27083 11 24653 38 .04514 12 29514 39 .13542 13 11944 40 .08681 14 .05208 41 40347 15 12500 42 12639 16 25000 43 .18403 17 40069 44 .70833 18 .02500 45 .15625 19 12014 46 .24653 20 .11458 47 .04514 21 00347 48 .01736 22 .12014 49 .08889 23 .04861 50 .05208 24 .02778 51 .02778 25 32639 52 .03472 26 .64931 53 .23611 27 .14931 54 35972 2.